Difference between revisions of "Manuals/calci/MOORE"
Jump to navigation
Jump to search
| Line 9: | Line 9: | ||
*The Moore matrix has successive powers of the applied to the first column, so it is an mxn matrix of the form: | *The Moore matrix has successive powers of the applied to the first column, so it is an mxn matrix of the form: | ||
<math>\begin{bmatrix} | <math>\begin{bmatrix} | ||
| − | \alpha_1 & {\alpha_1}^ | + | \alpha_1 & {\alpha_1}^q &\cdots & {\alpha_1}^q^{n-1} \\ |
| − | \alpha_2 & {\alpha_2}^ | + | \alpha_2 & {\alpha_2}^q &\cdots & {\alpha_2}^q^{n-1} \\ |
| − | \alpha_3 & {\alpha_3}^ | + | \alpha_3 & {\alpha_3}^q &\cdots & {\alpha_3}^q^{n-1} \\ |
\vdots & \ddots & \vdots \\ | \vdots & \ddots & \vdots \\ | ||
| − | \alpha_m & {\alpha_m}^ | + | \alpha_m & {\alpha_m}^q &\cdots & {\alpha_m}^q^{n-1} \\ |
\end{bmatrix} </math> | \end{bmatrix} </math> | ||
*In calci, MATRIX("moore") is giving the matrixwith the element 1 of order 3. | *In calci, MATRIX("moore") is giving the matrixwith the element 1 of order 3. | ||
*And MATRIX("moore",4,1..4) is giving Moore matrix starting element 1 to 4 of order 4. | *And MATRIX("moore",4,1..4) is giving Moore matrix starting element 1 to 4 of order 4. | ||
Revision as of 09:57, 30 April 2015
MATRIX("MOORE",order)
- is the size of the Moore matrix.
Description
- This function gives the moore matrix of order 3 with the element 1.
- A moore matrix, is a square matrix over a finite field.
- When moore matrix is a square matrix, then its deteminant is called a Moore determinant.
- But it is unrelated to the Moore determinant of a quaternionic Hermitian matrix.
- The Moore matrix has successive powers of the applied to the first column, so it is an mxn matrix of the form:
Failed to parse (Conversion error. Server ("https://wikimedia.org/api/rest_") reported: "Cannot get mml. TeX parse error: Double exponent: use braces to clarify"): {\displaystyle {\begin{bmatrix}\alpha _{1}&{\alpha _{1}}^{q}&\cdots &{\alpha _{1}}^{q}^{n-1}\\\alpha _{2}&{\alpha _{2}}^{q}&\cdots &{\alpha _{2}}^{q}^{n-1}\\\alpha _{3}&{\alpha _{3}}^{q}&\cdots &{\alpha _{3}}^{q}^{n-1}\\\vdots &\ddots &\vdots \\\alpha _{m}&{\alpha _{m}}^{q}&\cdots &{\alpha _{m}}^{q}^{n-1}\\\end{bmatrix}}}
- In calci, MATRIX("moore") is giving the matrixwith the element 1 of order 3.
- And MATRIX("moore",4,1..4) is giving Moore matrix starting element 1 to 4 of order 4.
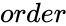 is the size of the Moore matrix.
is the size of the Moore matrix.