Difference between revisions of "Manuals/calci/SECH"
Jump to navigation
Jump to search
| Line 7: | Line 7: | ||
* SECH is the reciprocal of COSH function. | * SECH is the reciprocal of COSH function. | ||
* SECH(z)=<math>cosh (z)^{-1}</math> i.e, <math>\frac{ 2} {e^z+e^{-z}} </math> or SEC(iz). where 'i' is the imaginary unit and <math>i=\sqrt{-1}</math> | * SECH(z)=<math>cosh (z)^{-1}</math> i.e, <math>\frac{ 2} {e^z+e^{-z}} </math> or SEC(iz). where 'i' is the imaginary unit and <math>i=\sqrt{-1}</math> | ||
| − | * Also relation between Hyperbolic & | + | * Also relation between Hyperbolic & Trigonometric function is <math>Sec(iz) = Sech(z)</math> & <math>Sec(iz) = Sec(z)</math> |
| + | *SECH(-z) = SECH(z) | ||
== Examples == | == Examples == | ||
Revision as of 06:05, 5 November 2013
SECH(z)
- where z is any real number
Description
- This function gives the hyperbolic Secant of 'z',
- It is also called as Circular function.
- SECH is the reciprocal of COSH function.
- SECH(z)= i.e, or SEC(iz). where 'i' is the imaginary unit and
- Also relation between Hyperbolic & Trigonometric function is &
- SECH(-z) = SECH(z)
Examples
SECH(z)
- z is any real number.
| SECH(z) | Value(Radian) |
| SECH(0) | 1 |
| SECH(10) | 0.00009079985933781728 |
| SECH(7) | SECH(7)=0.001823762414 |
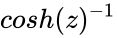 i.e,
i.e, 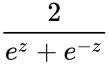 or SEC(iz). where 'i' is the imaginary unit and
or SEC(iz). where 'i' is the imaginary unit and 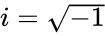
 &
& 