Difference between revisions of "Manuals/calci/VARP"
Jump to navigation
Jump to search
(Created page with "<div id="6SpaceContent" class="zcontent" align="left"> <font color="#484848"><font face="Arial, sans-serif"><font size="2">'''VARP'''</font></font></font><font color="#48484...") |
|||
| Line 1: | Line 1: | ||
| − | <div | + | <div style="font-size:30px">'''VAR(n1,n2,n3…)'''</div><br/> |
| + | *<math>n1,n2,n3,... </math> are numbers. | ||
| − | < | + | ==Description== |
| + | *This function gives the variance based on the entire population. | ||
| + | *Variance is a measure of dispersion obtained by taking the mean of the squared deviations of the observed values from their mean in a frequency distribution. | ||
| + | *i.e.,variance is a measure of how far each value in the data set is from the mean. | ||
| + | *It is denoted by <math> \sigma </math>. The square root of variance is called the standard deviation. | ||
| + | *In <math>VAR(n1,n2,n3,...)</math>, <math>n1,n2,n3,...</math> are numbers based on a population. Here <math>n1</math> is required. <math>n2,n3,...</math> are optional. | ||
| + | *To find the variance we can use the following formula: | ||
| + | <math>Variance= \frac{\sum (x_i-\bar{x})^2}{n-1}</math> | ||
| + | where <math> \bar{x}</math> is the sample mean of xi and n is the sample size. | ||
| + | *Suppose <math>\sigma = 0</math> which is indicating all the values are identical. | ||
| + | *When <math>\sigma </math> is non-zero then it is always positive. | ||
| + | *This function is considering our given data is the entire population. | ||
| + | *Suppose it should consider the data as the sample of the population, we can use the [[Manuals/calci/VAR | VAR ]] function. | ||
| + | *The arguments can be either numbers or names, array,constants or references that contain numbers. | ||
| + | *Suppose the array contains text,logical values or empty cells, like that values are not considered. | ||
| + | *When we are entering logical values and text representations of numbers as directly, then the arguments are counted. | ||
| + | *Suppose the function have to consider the logical values and text representations of numbers in a reference , we can use the [[Manuals/calci/VARPA | VARPA ]] function. | ||
| + | *This function will return the result as error when | ||
| + | 1. Any one of the argument is non-numeric. | ||
| + | 2. The arguments containing the error values or text that cannot be translated in to numbers. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ==Examples== | |
| − | + | {| class="wikitable" | |
| − | + | |+Spreadsheet | |
| − | + | |- | |
| − | + | ! !! A !! B !! C !! D!! E !! F | |
| − | + | |- | |
| − | + | ! 1 | |
| − | + | | 40 || 45 || 60 || 24 || 72 || 81 | |
| − | + | |- | |
| − | + | ! 2 | |
| − | + | | 10.21 || 11.65 || 17.81 || 15.02 || 18.18 || 27.41 | |
| − | + | |} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | #=VARP(A1:F1) = 377.555555556 | |
| + | #=VARP(A1:F2) = 31.4284222222 | ||
| + | #=VARP(30,32,37,41,TRUE) = 199.76 | ||
| + | #=VARP(40,61,53,46,FALSE) = 549.2 | ||
| − | + | ==See Also== | |
| − | + | *[[Manuals/calci/ DVAR | DVAR]] | |
| − | + | *[[Manuals/calci/DVARP | DVARP ]] | |
| − | + | *[[Manuals/calci/VARPA | VARPA ]] | |
| − | + | *[[Manuals/calci/VAR | VAR]] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ==References== | |
| − | |||
Revision as of 06:16, 7 February 2014
VAR(n1,n2,n3…)
- are numbers.
Description
- This function gives the variance based on the entire population.
- Variance is a measure of dispersion obtained by taking the mean of the squared deviations of the observed values from their mean in a frequency distribution.
- i.e.,variance is a measure of how far each value in the data set is from the mean.
- It is denoted by . The square root of variance is called the standard deviation.
- In , are numbers based on a population. Here is required. are optional.
- To find the variance we can use the following formula:
where is the sample mean of xi and n is the sample size.
- Suppose which is indicating all the values are identical.
- When is non-zero then it is always positive.
- This function is considering our given data is the entire population.
- Suppose it should consider the data as the sample of the population, we can use the VAR function.
- The arguments can be either numbers or names, array,constants or references that contain numbers.
- Suppose the array contains text,logical values or empty cells, like that values are not considered.
- When we are entering logical values and text representations of numbers as directly, then the arguments are counted.
- Suppose the function have to consider the logical values and text representations of numbers in a reference , we can use the VARPA function.
- This function will return the result as error when
1. Any one of the argument is non-numeric.
2. The arguments containing the error values or text that cannot be translated in to numbers.
Examples
| A | B | C | D | E | F | |
|---|---|---|---|---|---|---|
| 1 | 40 | 45 | 60 | 24 | 72 | 81 |
| 2 | 10.21 | 11.65 | 17.81 | 15.02 | 18.18 | 27.41 |
- =VARP(A1:F1) = 377.555555556
- =VARP(A1:F2) = 31.4284222222
- =VARP(30,32,37,41,TRUE) = 199.76
- =VARP(40,61,53,46,FALSE) = 549.2
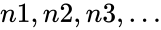 are numbers.
are numbers.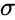 . The square root of variance is called the standard deviation.
. The square root of variance is called the standard deviation.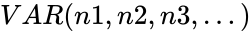 ,
,  is required.
is required. 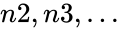 are optional.
are optional. where
where 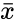 is the sample mean of xi and n is the sample size.
is the sample mean of xi and n is the sample size.
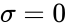 which is indicating all the values are identical.
which is indicating all the values are identical.