Difference between revisions of "Manuals/calci/PERMUTATION"
Jump to navigation
Jump to search
(Created page with "<div style="font-size:30px">'''PERMUTATION'''</div><br/>") |
|||
| Line 1: | Line 1: | ||
| − | <div style="font-size:30px">'''PERMUTATION'''</div><br/> | + | <div style="font-size:30px">'''MATRIX("PERMUTATION",order)'''</div><br/> |
| + | *<math>order</math> is the size of the Permutation matrix. | ||
| + | |||
| + | ==Description== | ||
| + | *This function returns the matrix Permutation matrix of order 3. | ||
| + | *A permutation matrix is a square binary matrix obtained by permuting the rows of an nxn identity matrix according to some permutation of the numbers 1 to n. | ||
| + | *This matrix has exactly one entry 1 in each row and each column and 0's elsewhere. | ||
| + | *A permutation matrix is nonsingular, and its determiant + or -. | ||
| + | *Also permutation matrix A having the following properties <math>AA^(T)=I</math>, where <math>A^(T)</math> is a transpose and I is the identity matrix. | ||
| + | *Permutation matrices are orthogonal (hence, their inverse is their transpose: <math>P^{-1} = P^T</math>). | ||
| + | *A permutation matrix allows to exchange rows or columns of another via the matrix-matrix product. | ||
| + | *In calci MATRIX("permutation",4) gives the permutation matrix of order 4. | ||
Revision as of 12:49, 30 April 2015
MATRIX("PERMUTATION",order)
- is the size of the Permutation matrix.
Description
- This function returns the matrix Permutation matrix of order 3.
- A permutation matrix is a square binary matrix obtained by permuting the rows of an nxn identity matrix according to some permutation of the numbers 1 to n.
- This matrix has exactly one entry 1 in each row and each column and 0's elsewhere.
- A permutation matrix is nonsingular, and its determiant + or -.
- Also permutation matrix A having the following properties , where is a transpose and I is the identity matrix.
- Permutation matrices are orthogonal (hence, their inverse is their transpose: ).
- A permutation matrix allows to exchange rows or columns of another via the matrix-matrix product.
- In calci MATRIX("permutation",4) gives the permutation matrix of order 4.
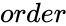 is the size of the Permutation matrix.
is the size of the Permutation matrix.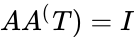 , where
, where 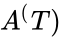 is a transpose and I is the identity matrix.
is a transpose and I is the identity matrix.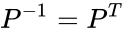 ).
).