Difference between revisions of "Manuals/calci/FROBENIUS"
Jump to navigation
Jump to search
(Created page with "<div style="font-size:30px">'''FROBENIUS'''</div><br/>") |
|||
| (4 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | <div style="font-size:30px">'''FROBENIUS'''</div><br/> | + | <div style="font-size:30px">'''MATRIX("FROBENIUS",order)'''</div><br/> |
| + | *<math>order</math> is the order of the matrix. | ||
| + | |||
| + | ==Description== | ||
| + | *This function gives the matrix with the property of Frobenius. | ||
| + | *A Frobenius matrix is a special kind of square matrix from numerical mathematics. | ||
| + | *A square matrix is a Frobenius matrix if it has the following three properties: | ||
| + | # All entries on the main diagonal are ones | ||
| + | # The entries below the main diagonal of at most one column are arbitrary | ||
| + | # Every other entry is zero. | ||
| + | *And also Frobenius matrices are invertible. | ||
| + | *The inverse of a Frobenius matrix is again a Frobenius matrix, equal to the original matrix with changed signs outside the main diagonal. | ||
| + | *Here MATRIX("frobenius") gives the frobenius matrix of order 3. | ||
| + | * In calci, users can get a different order of matrices also. | ||
| + | |||
| + | ==Examples== | ||
| + | #MATRIX("frobenius") = 1 | ||
| + | #MATRIX("frobenius",3) | ||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | 1 || 0 || 0 | ||
| + | |- | ||
| + | | 0 || 1 || 0 | ||
| + | |- | ||
| + | | 0 || 0.7699975343421102 || 1 | ||
| + | |} | ||
| + | |||
| + | ==Related Videos== | ||
| + | |||
| + | {{#ev:youtube|i0JGx8PEQW8|280|center|Norm of Matrix}} | ||
| + | |||
| + | ==See Also== | ||
| + | *[[Manuals/calci/ANTIDIAGONAL| ANTIDIAGONAL]] | ||
| + | *[[Manuals/calci/CONFERENCE| CONFERENCE]] | ||
| + | *[[Manuals/calci/CIRCULANT| CIRCULANT]] | ||
| + | *[[Manuals/calci/HANKEL| HANKEL]] | ||
| + | |||
| + | ==References== | ||
| + | *[http://en.wikipedia.org/wiki/Frobenius_matrix Frobenius matrix] | ||
Latest revision as of 00:53, 26 October 2015
MATRIX("FROBENIUS",order)
- is the order of the matrix.
Description
- This function gives the matrix with the property of Frobenius.
- A Frobenius matrix is a special kind of square matrix from numerical mathematics.
- A square matrix is a Frobenius matrix if it has the following three properties:
- All entries on the main diagonal are ones
- The entries below the main diagonal of at most one column are arbitrary
- Every other entry is zero.
- And also Frobenius matrices are invertible.
- The inverse of a Frobenius matrix is again a Frobenius matrix, equal to the original matrix with changed signs outside the main diagonal.
- Here MATRIX("frobenius") gives the frobenius matrix of order 3.
- In calci, users can get a different order of matrices also.
Examples
- MATRIX("frobenius") = 1
- MATRIX("frobenius",3)
| 1 | 0 | 0 |
| 0 | 1 | 0 |
| 0 | 0.7699975343421102 | 1 |
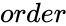 is the order of the matrix.
is the order of the matrix.