Difference between revisions of "Manuals/calci/HERMITIAN"
Jump to navigation
Jump to search
| (9 intermediate revisions by 2 users not shown) | |||
| Line 5: | Line 5: | ||
*This function gives the Hermitian matrix of order 3. | *This function gives the Hermitian matrix of order 3. | ||
*A Hermitian matrix is defined as the square matrix with complex entries which is equal to its own conjugate transpose. | *A Hermitian matrix is defined as the square matrix with complex entries which is equal to its own conjugate transpose. | ||
| − | *i.e., the matrix A is Hermitian if and only if A=A^T, where A^T denotes the conjugate transpose, which is equivalent to the condition a_ | + | *i.e., the matrix A is Hermitian if and only if <math>A=A^T</math>, where <math>A^T</math> denotes the conjugate transpose, which is equivalent to the condition <math> a_{ij}=\bar {a^{ji}}</math>. |
| − | *A | + | *A hermitian matrix is also called as self-adjoint matrix. |
*The following matrix is the example of 3x3 Hermitian matrix: | *The following matrix is the example of 3x3 Hermitian matrix: | ||
<math>\begin{bmatrix} | <math>\begin{bmatrix} | ||
| Line 15: | Line 15: | ||
*The diagonal elements must be real, as they must be their own complex conjugate. | *The diagonal elements must be real, as they must be their own complex conjugate. | ||
*An integer or real matrix is Hermitian iff it is symmetric. | *An integer or real matrix is Hermitian iff it is symmetric. | ||
| − | *In calci, users can change the order and number of the Hermitian matrices. | + | *In calci, users can change the order and number of the Hermitian matrices. |
==Examples== | ==Examples== | ||
| − | *1.MATRIX("hermitian") | + | *1.MATRIX("hermitian") = -72 |
| + | *2.MATRIX("hermitian",3) | ||
{| class="wikitable" | {| class="wikitable" | ||
|- | |- | ||
| Line 27: | Line 28: | ||
| 49 + 40i || 0 + -34i || -33 | | 49 + 40i || 0 + -34i || -33 | ||
|} | |} | ||
| − | * | + | *3.MATRIX("hermitian",5) |
{| class="wikitable" | {| class="wikitable" | ||
|- | |- | ||
| Line 40: | Line 41: | ||
| -13 + 21i || 83 + 95i || 88 + 81i || -70 + 92i || -7 | | -13 + 21i || 83 + 95i || 88 + 81i || -70 + 92i || -7 | ||
|} | |} | ||
| + | |||
| + | ==Related Videos== | ||
| + | |||
| + | {{#ev:youtube|dEKS8ou6F7k|280|center|Hermitian Matrix}} | ||
==See Also== | ==See Also== | ||
| Line 48: | Line 53: | ||
==References== | ==References== | ||
| + | *[http://en.wikipedia.org/wiki/Hermitian_matrix Hermitian matrix] | ||
Latest revision as of 01:10, 26 October 2015
MATRIX("HERMITIAN",order)
- is the order of the Hermitian matrix.
Description
- This function gives the Hermitian matrix of order 3.
- A Hermitian matrix is defined as the square matrix with complex entries which is equal to its own conjugate transpose.
- i.e., the matrix A is Hermitian if and only if , where denotes the conjugate transpose, which is equivalent to the condition .
- A hermitian matrix is also called as self-adjoint matrix.
- The following matrix is the example of 3x3 Hermitian matrix:
.
- The diagonal elements must be real, as they must be their own complex conjugate.
- An integer or real matrix is Hermitian iff it is symmetric.
- In calci, users can change the order and number of the Hermitian matrices.
Examples
- 1.MATRIX("hermitian") = -72
- 2.MATRIX("hermitian",3)
| -62 | -48 + 4i | 49 + -40i |
| -48 + -4i | -54 | 0 + 34i |
| 49 + 40i | 0 + -34i | -33 |
- 3.MATRIX("hermitian",5)
| -90 | -75 + 79i | 56 + -17i | 92 + -51i | -13 + -21i |
| -75 + -79i | -19 | -77 + -19i | 42 + 47i | 83 + -95i |
| 56 + 17i | -77 + 19i | -60 | -25 + -26i | 88 + -81i |
| 92 + 51i | 42 + -47i | -25 + 26i | -89 | -70 + -92i |
| -13 + 21i | 83 + 95i | 88 + 81i | -70 + 92i | -7 |
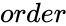 is the order of the Hermitian matrix.
is the order of the Hermitian matrix. , where
, where 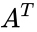 denotes the conjugate transpose, which is equivalent to the condition
denotes the conjugate transpose, which is equivalent to the condition 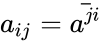 .
. .
.