Difference between revisions of "Manuals/calci/CARTESIANPRODUCT"
Jump to navigation
Jump to search
(Created page with "CARTESIANPRODUCT") |
|||
| Line 1: | Line 1: | ||
| − | CARTESIANPRODUCT | + | <div style="font-size:30px">''' CARTESIANPRODUCT (GivenSet1,GivenSet2) '''</div><br/> |
| + | *<math>GivenSet1</math> and <math>GivenSet2</math> are the set of numbers to find product. | ||
| + | |||
| + | ==Description== | ||
| + | *This function shows the Cartesian product of two sets. | ||
| + | *Cartesian product is the product of two sets. | ||
| + | *The product of set X and set Y is the set that contains all ordered pairs ( x, y ) for which x belongs to X and y belongs to Y. | ||
| + | *In <math>CARTESIANPRODUCT(GivenSet1,GivenSet2)</math>,<math>Givenset1</math> and <math>Givenset2</math> are two set of real numbers with a pair of numbers. | ||
| + | *Consider two sets A and B. | ||
| + | *The Cartesian product of A and B are denoted by s the set of all ordered pairs (a,b) such that a Belongs to A and b Belongs to B. | ||
| + | <math>\llcorner A </math> | ||
| + | ==References== | ||
| + | [http://ndp.jct.ac.il/tutorials/discrete/node28.html Cartesian Product] | ||
Revision as of 18:13, 21 December 2016
CARTESIANPRODUCT (GivenSet1,GivenSet2)
- and are the set of numbers to find product.
Description
- This function shows the Cartesian product of two sets.
- Cartesian product is the product of two sets.
- The product of set X and set Y is the set that contains all ordered pairs ( x, y ) for which x belongs to X and y belongs to Y.
- In , and are two set of real numbers with a pair of numbers.
- Consider two sets A and B.
- The Cartesian product of A and B are denoted by s the set of all ordered pairs (a,b) such that a Belongs to A and b Belongs to B.
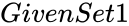 and
and  are the set of numbers to find product.
are the set of numbers to find product. ,
,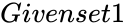 and
and  are two set of real numbers with a pair of numbers.
are two set of real numbers with a pair of numbers.