Difference between revisions of "Manuals/calci/SKEW"
Jump to navigation
Jump to search
| (4 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | <div style="font-size:30px">'''SKEW( | + | <div style="font-size:30px">'''SKEW()'''</div><br/> |
| − | * | + | *Parameters are any numbers to calculate the skewness. |
| + | **SKEW() returns the skewness of a distribution | ||
==Description== | ==Description== | ||
| Line 11: | Line 12: | ||
*In a Normal Skewed Distribution, its <math>mean=median=mode</math> | *In a Normal Skewed Distribution, its <math>mean=median=mode</math> | ||
*In a Right Skewed Distribution, its <math>mode<median<mean</math>. | *In a Right Skewed Distribution, its <math>mode<median<mean</math>. | ||
| − | *In <math>SKEW( | + | *In <math>SKEW(), First parameter is required.From the second parameter are optional. |
*In calci there is no restriction for giving the number of arguments. | *In calci there is no restriction for giving the number of arguments. | ||
*The arguments can be be either numbers or names, array,constants or references that contain numbers. | *The arguments can be be either numbers or names, array,constants or references that contain numbers. | ||
| Line 63: | Line 64: | ||
==References== | ==References== | ||
*[http://en.wikipedia.org/wiki/Skewness Skewness] | *[http://en.wikipedia.org/wiki/Skewness Skewness] | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | *[[Z_API_Functions | List of Main Z Functions]] | ||
| + | |||
| + | *[[ Z3 | Z3 home ]] | ||
Latest revision as of 13:40, 18 June 2018
SKEW()
- Parameters are any numbers to calculate the skewness.
- SKEW() returns the skewness of a distribution
Description
- This function gives the Skewness of a distribution.
- Skewness is a measure of the degree of asymmetry of a distribution.
- A distribution(normal distribution) is symmetry ,it don't have a Skewness.
- In a distribution the left tail is more pronounced than the right tail (towards more negative values) then the function is said to have Negative Skewness.
- If a distribution is skewed to the right, the tail on the curve's right-hand side is longer than the tail on the left-hand side (towards more positive values), then the function is said to have a positive skewness.
- In a Left Skewed Distribution, its
- In a Normal Skewed Distribution, its
- In a Right Skewed Distribution, its .
- In
Where, is the sample standard deviation, represents a sample mean.
- This function will return the result as error when
1. Any one of the argument is non-numeric. 2. If there are fewer than three data points, or the Sample Standard Deviation is zero.
Examples
| A | B | C | D | E | |
|---|---|---|---|---|---|
| 1 | 0 | 4 | -5 | 4 | 1 |
| 2 | 29 | 9 | 11 | 5 | 2 |
| 3 | 41 | 11 | 18 | 2 | 3 |
| 4 | 18 | 10 | 7 | 5 | 5 |
| 5 | 4 | 5 | 9 | 6 | 6 |
| 6 | 38 | 9 | 13 | 8 | 11 |
- =SKEW(B1:B5) = -0.4369344921493
- =SKEW(A1:A6) = -0.21921252920
- =SKEW(C1:C4) = -0.715957010
- =SKEW(D1:D6) = 0
- =SKEW(E1:E6) = 1.16584702768
Related Videos
See Also
References
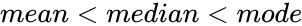
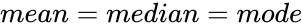
 .
.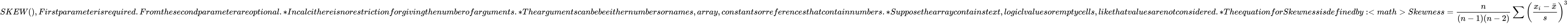
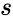 is the sample standard deviation,
is the sample standard deviation, 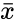 represents a sample mean.
represents a sample mean.