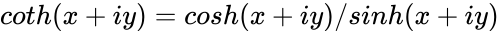Difference between revisions of "Manuals/calci/IMCOTH"
Jump to navigation
Jump to search
(Created page with "<div style="font-size:30px">'''IMCOTH(iz)'''</div><br/> * where iz is the complex number ==Description== *This function gives the hyperbolic cotangent (coth) value of a comp...") |
|||
| Line 1: | Line 1: | ||
| − | <div style="font-size:30px">'''IMCOTH( | + | <div style="font-size:30px">'''IMCOTH(ComplexNumber)'''</div><br/> |
| − | * where | + | * where <math>ComplexNumber</math> is any complex number. |
==Description== | ==Description== | ||
*This function gives the hyperbolic cotangent (coth) value of a complex number. | *This function gives the hyperbolic cotangent (coth) value of a complex number. | ||
| − | * | + | *Consider the complex number is the form of <math>x+iy</math> |
*x & y are the real numbers. | *x & y are the real numbers. | ||
*'i' is the imaginary unit <math>i=\sqrt{-1}</math> | *'i' is the imaginary unit <math>i=\sqrt{-1}</math> | ||
| Line 13: | Line 13: | ||
== Examples == | == Examples == | ||
| − | '''IMCOTH( | + | '''IMCOTH(ComplexNumber)''' |
| − | *''' | + | *'''ComplexNumber''' is any complex number. |
{|id="TABLE1" class="SpreadSheet blue" | {|id="TABLE1" class="SpreadSheet blue" | ||
|- class="even" | |- class="even" | ||
| − | |'''IMCOTH( | + | |'''IMCOTH(ComplexNumber)''' |
|'''Value''' | |'''Value''' | ||
Revision as of 15:08, 16 July 2018
IMCOTH(ComplexNumber)
- where is any complex number.
Description
- This function gives the hyperbolic cotangent (coth) value of a complex number.
- Consider the complex number is the form of
- x & y are the real numbers.
- 'i' is the imaginary unit
- Also x is called the real part & y is the imaginary part of a complex number.
- COMPLEX is the function used to convert Real & Imaginary numbers in to a complex number.
- is defined by
Examples
IMCOTH(ComplexNumber)
- ComplexNumber is any complex number.
| IMCOTH(ComplexNumber) | Value |
| IMCOTH("7+4i") | 0.9999997580237623-i0.0000016453591929519932 |
| IMCOTH("7-8i") | 0.9999984073584316-i7 |
| IMCOTH("3") | 1.004969823313689+i0 |
Related Videos
See Also
References
 is any complex number.
is any complex number.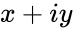
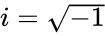
 is defined by
is defined by