Difference between revisions of "Manuals/calci/ANOVATWOFACTORWITHREPLICATION"
Jump to navigation
Jump to search
| (2 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | =ANOVATWOFACTORWITHREPLICATION(Array, Alpha, | + | <div style="font-size:30px">'''ANOVATWOFACTORWITHREPLICATION (Array,Alpha,NumberofSamplesPerRow,NewTableFlag) '''</div><br/> |
*where, <math>Array</math> is an array of numbers or reference to cells containing array | *where, <math>Array</math> is an array of numbers or reference to cells containing array | ||
| Line 5: | Line 5: | ||
*<math>NumberOfSamples</math> represents the number of samples in an array | *<math>NumberOfSamples</math> represents the number of samples in an array | ||
*<math>NewTableFlag</math> that decides to display the output in the same worksheet or a new space cube. | *<math>NewTableFlag</math> that decides to display the output in the same worksheet or a new space cube. | ||
| − | + | **ANOVATWOFACTORWITHREPLICATION(), performs an analysis of variances between more more data sets.This should be used when you have more than one sample from each data set. | |
| − | ANOVATWOFACTORWITHREPLICATION() performs an analysis of variances between | ||
== Description == | == Description == | ||
| Line 162: | Line 161: | ||
| 23 | | 23 | ||
|} | |} | ||
| + | |||
| + | ==Related Videos== | ||
| + | |||
| + | {{#ev:youtube|j9ZPMlVHJVs|280|center|ANOVA}} | ||
== See Also == | == See Also == | ||
| Line 172: | Line 175: | ||
*[http://en.wikipedia.org/wiki/Analysis_of_variance ANOVA] | *[http://en.wikipedia.org/wiki/Analysis_of_variance ANOVA] | ||
*[http://en.wikipedia.org/wiki/Analysis_of_variance#ANOVA_for_multiple_factors Anova Multiple Factors] | *[http://en.wikipedia.org/wiki/Analysis_of_variance#ANOVA_for_multiple_factors Anova Multiple Factors] | ||
| + | |||
| + | |||
| + | |||
| + | *[[Z_API_Functions | List of Main Z Functions]] | ||
| + | |||
| + | *[[ Z3 | Z3 home ]] | ||
Latest revision as of 15:56, 17 August 2018
ANOVATWOFACTORWITHREPLICATION (Array,Alpha,NumberofSamplesPerRow,NewTableFlag)
- where, is an array of numbers or reference to cells containing array
- represents a constant value between '0' and '1'
- represents the number of samples in an array
- that decides to display the output in the same worksheet or a new space cube.
- ANOVATWOFACTORWITHREPLICATION(), performs an analysis of variances between more more data sets.This should be used when you have more than one sample from each data set.
Description
ANOVATWOFACTORWITHREPLICATION(Array, Alpha, NumberOfSamples, NewTableFlag)
- Analysis Of Variances(ANOVA) is a method of checking relationship between two or more data sets.
- should be in between '0' and '1'. Else Calci displays #N/A error message.
- Data in an array can be divided in one or more groups. That is represented by .
- can be a logical value TRUE or FALSE. If omitted, Calci assumes it to be FALSE.
- If is TRUE, the result is displayed on new zspace sheet.
Examples
Consider the following table with Columns A and B as array inputs for ANOVATWOFACTORWITHREPLICATION() function.
| 1 | 3 | |
| 2 | 5 | |
| 6 | 41 | |
| 8 | 45 | |
| 9 | 56 | |
| 10 | 8 | |
| 7 | 8 | |
| 12 | 5 | |
| 17 | 18 | |
| 72 | 31 | |
| 6 | 3 | |
| 2 | 7 | |
=ANOVATWOFACTORWITHREPLICATION(A1:B12, 0.01, 2, TRUE) returns the result in new Zspace cube.
| Groups | Count | Sum | Average | Variance |
|---|---|---|---|---|
| Array1 | 12 | 152 | 12.666666666666666 | 369.69696969696975 |
| Array2 | 12 | 230 | 19.166666666666668 | 360.3333333333333 |
| Source of Variation | Sum of Squares | Degree of Freedom | Mean Square | F-Value | Probability | F Critical |
|---|---|---|---|---|---|---|
| Sample: | 3270.333333333334 | 5 | 654.0666666666668 | 2.8081574239713785 | 0.06629115945265437 | 5.064312767657053 |
| Columns: | 253.50000000000017 | 1 | 253.50000000000017 | 1.0883720930232565 | 0.36437615127626016 | 9.330229140735954 |
| Interaction: | 1965.0000000000018 | 5 | 393.00000000000034 | 1.6872987477638656 | 0.2120282015971952 | 5.0650509516310676 |
| Within: | 2795 | 12 | 232.91666666666666 | |||
| Total: | 8283.833333333336 | 23 |
Related Videos
See Also
References
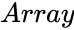 is an array of numbers or reference to cells containing array
is an array of numbers or reference to cells containing array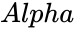 represents a constant value between '0' and '1'
represents a constant value between '0' and '1'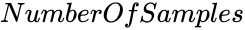 represents the number of samples in an array
represents the number of samples in an array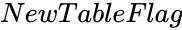 that decides to display the output in the same worksheet or a new space cube.
that decides to display the output in the same worksheet or a new space cube.