Difference between revisions of "Manuals/calci/QRDECOMPOSITION"
Jump to navigation
Jump to search
| (16 intermediate revisions by 2 users not shown) | |||
| Line 4: | Line 4: | ||
==Description== | ==Description== | ||
*This function gives the value of QR Decomposition. | *This function gives the value of QR Decomposition. | ||
| − | *In <math>QRDECOMPOSITION (Matrix)</math>,<math>Matrix</math> is any matrix. | + | *In <math>QRDECOMPOSITION (Matrix)</math>, <math>Matrix</math> is any matrix. |
*QR Decomposition is also called QR Factorization. | *QR Decomposition is also called QR Factorization. | ||
*QR Decomposition is defined by the product of Orthogonal matrix and Upper Triangular matrix. | *QR Decomposition is defined by the product of Orthogonal matrix and Upper Triangular matrix. | ||
| − | *Consider any square matrix A may be decomposed as <math>A=QR</math>,where <math>Q</math> stands for orthogonal matrix and <math>R</math> stands for Upper Triangular matrix. | + | *Consider any square matrix A may be decomposed as <math>A=QR</math>, where <math>Q</math> stands for orthogonal matrix and <math>R</math> stands for Upper Triangular matrix. |
| − | *An orthogonal matrix should satisfy <math>Q^TQ=I</math>,where <math>I</math> is identity or Unitary matrix. | + | *An orthogonal matrix should satisfy <math>Q^TQ=I</math>, where <math>I</math> is identity or Unitary matrix. |
*<math>Q^T</math> is the transpose matrix of Q. | *<math>Q^T</math> is the transpose matrix of Q. | ||
| − | *If the given matrix A is non singular, then this factorization is unique. | + | *If the given matrix A is non-singular, then this factorization is unique. |
*Gram-Schmidt process is one of the process of computing decomposition in QR Decomposition method. | *Gram-Schmidt process is one of the process of computing decomposition in QR Decomposition method. | ||
==Examples== | ==Examples== | ||
| − | 1 | + | {| class="wikitable" |
| + | |+Spreadsheet | ||
| + | |- | ||
| + | ! !! A !! B | ||
| + | |- | ||
| + | ! 1 | ||
| + | | 2 || 6 | ||
| + | |- | ||
| + | !2 | ||
| + | | 10 || -15 | ||
| + | |} | ||
| + | =QRDECOMPOSITION(A1:B2) | ||
{| border="1" cellpadding="5" cellspacing="0" | {| border="1" cellpadding="5" cellspacing="0" | ||
|- | |- | ||
| | | | ||
-0.19611613513818393 -0.9805806756909202 | -0.19611613513818393 -0.9805806756909202 | ||
| − | || -0.9805806756909202 0.19611613513818393 | + | || |
| + | -0.9805806756909202 0.19611613513818393 | ||
|- | |- | ||
| | | | ||
-10.19803902718557 13.5320133245347 | -10.19803902718557 13.5320133245347 | ||
| − | || -1.1102230246251565e-15 -8.825226081218279 | + | || |
| + | -1.1102230246251565e-15 -8.825226081218279 | ||
| + | |} | ||
| + | |||
| + | {| class="wikitable" | ||
| + | |+Spreadsheet | ||
| + | |- | ||
| + | ! !! A !! B !! C | ||
| + | |- | ||
| + | ! 1 | ||
| + | | 3 || 8 || -5 | ||
| + | |- | ||
| + | !2 | ||
| + | |4 || -6.3 || 9 | ||
| + | |- | ||
| + | !3 | ||
| + | |2 || 5 || -1 | ||
|} | |} | ||
| − | + | =QRDECOMPOSITION(A1:C3) | |
{| border="1" cellpadding="5" cellspacing="0" | {| border="1" cellpadding="5" cellspacing="0" | ||
|- | |- | ||
| | | | ||
-0.5570860145311556 0.631547425332445 -0.5392615524675877 | -0.5570860145311556 0.631547425332445 -0.5392615524675877 | ||
| − | || -0.7427813527082074 -0.669329688618384 -0.01654176541311622 | + | || |
| − | || -0.3713906763541037 0.3913382392381005 0.841975859527614 | + | -0.7427813527082074 -0.669329688618384 -0.01654176541311622 |
| + | || | ||
| + | -0.3713906763541037 0.3913382392381005 0.841975859527614 | ||
|- | |- | ||
| − | | -5.385164807134504 -1.634118975958056 -3.528211425363985 | + | | |
| + | -5.385164807134504 -1.634118975958056 -3.528211425363985 | ||
|| | || | ||
-2.1551618871879059e-16 11.22584763714588 -9.573042563465782 | -2.1551618871879059e-16 11.22584763714588 -9.573042563465782 | ||
| − | || | + | || |
5.3446973501217775e-17 0 1.7054560140922779 | 5.3446973501217775e-17 0 1.7054560140922779 | ||
|} | |} | ||
| + | |||
| + | ==Related Videos== | ||
| + | |||
| + | {{#ev:youtube|v=J41Ypt6Mftc|280|center|QR Decomposition}} | ||
| + | |||
| + | |||
| + | ==See Also== | ||
| + | *[[Manuals/calci/LUDECOMPOSITION| LUDECOMPOSITION]] | ||
| + | *[[Manuals/calci/CHOLESKYFACTORIZATION| CHOLESKYFACTORIZATION]] | ||
| + | *[[Manuals/calci/CONFERENCE| CONFERENCE]] | ||
| + | |||
| + | ==References== | ||
| + | *[https://en.wikipedia.org/wiki/LU_decomposition LU Decomposition] | ||
| + | |||
| + | *[[Z_API_Functions | List of Main Z Functions]] | ||
| + | |||
| + | *[[ Z3 | Z3 home ]] | ||
Latest revision as of 13:30, 2 May 2019
QRDECOMPOSITION (Matrix)
- is the set of values.
Description
- This function gives the value of QR Decomposition.
- In , is any matrix.
- QR Decomposition is also called QR Factorization.
- QR Decomposition is defined by the product of Orthogonal matrix and Upper Triangular matrix.
- Consider any square matrix A may be decomposed as , where stands for orthogonal matrix and stands for Upper Triangular matrix.
- An orthogonal matrix should satisfy , where is identity or Unitary matrix.
- is the transpose matrix of Q.
- If the given matrix A is non-singular, then this factorization is unique.
- Gram-Schmidt process is one of the process of computing decomposition in QR Decomposition method.
Examples
| A | B | |
|---|---|---|
| 1 | 2 | 6 |
| 2 | 10 | -15 |
=QRDECOMPOSITION(A1:B2)
-0.19611613513818393 -0.9805806756909202 |
-0.9805806756909202 0.19611613513818393 |
-10.19803902718557 13.5320133245347 |
-1.1102230246251565e-15 -8.825226081218279 |
| A | B | C | |
|---|---|---|---|
| 1 | 3 | 8 | -5 |
| 2 | 4 | -6.3 | 9 |
| 3 | 2 | 5 | -1 |
=QRDECOMPOSITION(A1:C3)
-0.5570860145311556 0.631547425332445 -0.5392615524675877 |
-0.7427813527082074 -0.669329688618384 -0.01654176541311622 |
-0.3713906763541037 0.3913382392381005 0.841975859527614 |
-5.385164807134504 -1.634118975958056 -3.528211425363985 |
-2.1551618871879059e-16 11.22584763714588 -9.573042563465782 |
5.3446973501217775e-17 0 1.7054560140922779 |
Related Videos
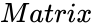 is the set of values.
is the set of values. ,
, 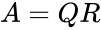 , where
, where  stands for orthogonal matrix and
stands for orthogonal matrix and 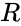 stands for Upper Triangular matrix.
stands for Upper Triangular matrix.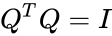 , where
, where 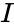 is identity or Unitary matrix.
is identity or Unitary matrix.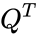 is the transpose matrix of Q.
is the transpose matrix of Q.