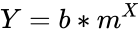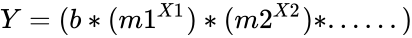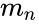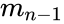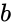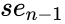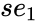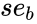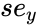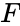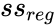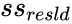Difference between revisions of "Manuals/calci/LOGEST"
Jump to navigation
Jump to search
| (One intermediate revision by the same user not shown) | |||
| Line 1: | Line 1: | ||
| − | |||
<div style="font-size:30px">'''LOGEST (YRange,XRange,Constant,Stats)'''</div><br/> | <div style="font-size:30px">'''LOGEST (YRange,XRange,Constant,Stats)'''</div><br/> | ||
| Line 7: | Line 6: | ||
*<math>Constant</math> is a logical value TRUE or FALSE, that decides whether to force the constant 'b' to 1, | *<math>Constant</math> is a logical value TRUE or FALSE, that decides whether to force the constant 'b' to 1, | ||
*<math>Stats</math> is a logical value TRUE or FALSE, that decides whether to return additional regression statistics. | *<math>Stats</math> is a logical value TRUE or FALSE, that decides whether to return additional regression statistics. | ||
| − | + | **LOGEST() is an array function that calculates the exponential curve that fits the data values and returns an array of values that describes the curve. | |
| − | LOGEST() is an array function that calculates the exponential curve that fits the data values and returns an array of values that describes the curve. | ||
== Description == | == Description == | ||
Latest revision as of 16:19, 22 August 2018
LOGEST (YRange,XRange,Constant,Stats)
where,
- is a set of Y values,
- is an optional set of X values,
- is a logical value TRUE or FALSE, that decides whether to force the constant 'b' to 1,
- is a logical value TRUE or FALSE, that decides whether to return additional regression statistics.
- LOGEST() is an array function that calculates the exponential curve that fits the data values and returns an array of values that describes the curve.
Description
- If 'YRange' is the set of dependent variable, 'XRange' is the set of independent variable, 'm' is a constant base for X value and 'b' is constant (Y-intercept),
then equation for curve is -
- For multiple ranges of X-values,
- Argument values and should be numeric, else Calci displays NaN error message.
- The length of array of XRange values should be equal to length of array of YRange values, else Calci displays #NULL error message.
- is a logical value that decides whether to make constant 'b' equal to 1.
- If = TRUE or omitted, 'b' is calculated normally. If = FALSE, 'b' is made equal to 1.
- is a logical value that decides whether to display additional regression statistics.
- If = TRUE, calci returns additional regresstion statistics. If = FALSE or omitted, Calci returns the values of 'm coefficients' and the constant 'b'.
- When there is only one independent X variable, Y intercept (b) can be calculated using following formulas -
- The additional regression is displayed in the following format where each statistic value is described as below-
| --- | ||||
| --- | ||||
- is an array of constant base values for curve equation
- is the constant value of Y when X=0
- is the standard error value for m1
- is the standard error value for constant b
- is the coefficient of determination
- is the standard error value for Y estimate
- is the observed F value
- is the number of degrees of freedom
- is the regression sum of squares
- is the residual sum of squares
Examples
| X1 values | X2 values | Y values | |
| 1 | 15 | 5 | |
| 2 | 17 | 9 | |
| 3 | 23 | 11 | |
| 4 | 28 | 16 | |
| 5 | 30 | 20 |
Example 1: With single range of X values -
=LOGEST(C2:C6,A2:A6,TRUE,TRUE) : Displays all the regression statistics for curve
with Y values in cells C2 to C6 and X values in cells A2 to A6.
| 1.3976542375431584 | 4.015612511401349 |
| 0.035964826100314505 | 0.11928183382512401 |
| 0.9665390759484563 | 0.11373076612886521 |
| 86.65681866342828 | 3 |
| 1.1208788400613339 | 0.038804061492775904 |
Example 2: With multiple range of X values -
=LOGEST(C2:C6,A2:B6,TRUE,TRUE) : Displays all the regression statistics for curve
with Y values in cells C2 to C6 and X values in cells A2 to B6.
| 0.9684996526566505 | 1.593646236498643 |
| 0.05737674420683413 | 0.23878654115432985 |
| 0.9710443899207976 | 0.12957493182116453 |
| 33.53562184546261 | 2 |
| 1.1261035756411908 | 0.03357932591291887 |
Related Videos
See Also
References
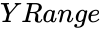 is a set of Y values,
is a set of Y values,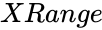 is an optional set of X values,
is an optional set of X values,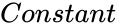 is a logical value TRUE or FALSE, that decides whether to force the constant 'b' to 1,
is a logical value TRUE or FALSE, that decides whether to force the constant 'b' to 1,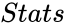 is a logical value TRUE or FALSE, that decides whether to return additional regression statistics.
is a logical value TRUE or FALSE, that decides whether to return additional regression statistics.