Difference between revisions of "Manuals/calci/DIVIDE"
Jump to navigation
Jump to search
| (3 intermediate revisions by the same user not shown) | |||
| Line 12: | Line 12: | ||
==Divisors== | ==Divisors== | ||
| − | *An integer <math>x</math> is called a divisor | + | *An integer <math>x</math> is called a divisor of the number <math>n</math> if dividing <math>n</math> by <math>x</math> leaves no reminder. |
| + | *Divisor of a number is also called a factor of a number. | ||
*DIVISORS(10) = 1 2 5 10 | *DIVISORS(10) = 1 2 5 10 | ||
*DIVISORS(1..10) | *DIVISORS(1..10) | ||
| Line 20: | Line 21: | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| + | |- class="odd" | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| class="sshl_f" | 2 | | class="sshl_f" | 2 | ||
| + | |- class="even" | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| class="sshl_f" | 3 | | class="sshl_f" | 3 | ||
| + | |- class="odd" | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| class="sshl_f" | 2 | | class="sshl_f" | 2 | ||
| class="sshl_f" | 4 | | class="sshl_f" | 4 | ||
| + | |- class="even" | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| class="sshl_f" | 5 | | class="sshl_f" | 5 | ||
| + | |- class="odd" | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| class="sshl_f" | 2 | | class="sshl_f" | 2 | ||
| Line 38: | Line 44: | ||
| class="sshl_f" | 6 | | class="sshl_f" | 6 | ||
| + | |- class="even" | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| class="sshl_f" | 7 | | class="sshl_f" | 7 | ||
| + | |- class="odd" | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| class="sshl_f" | 2 | | class="sshl_f" | 2 | ||
| Line 46: | Line 54: | ||
| class="sshl_f" | 8 | | class="sshl_f" | 8 | ||
| + | |- class="even" | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| class="sshl_f" | 3 | | class="sshl_f" | 3 | ||
| class="sshl_f" | 9 | | class="sshl_f" | 9 | ||
| + | |- class="odd" | ||
| class="sshl_f" | 1 | | class="sshl_f" | 1 | ||
| class="sshl_f" | 2 | | class="sshl_f" | 2 | ||
| Line 55: | Line 65: | ||
| class="sshl_f" | 10 | | class="sshl_f" | 10 | ||
|} | |} | ||
| + | |||
| + | ==Proper Divisor== | ||
| + | *The proper divisors of a positive integer N are those numbers, other than N itself, that divide N without remainder. | ||
| + | *For N > 1 they will always include 1, but for N = 1 there are no proper divisors. | ||
| + | *The proper divisors of 6 are 1, 2, and 3. | ||
| + | *PROPERDIVISORS(10) = 1 2 5 | ||
| + | *ALIQUOTDIVISORS(15) = 1 3 5 | ||
==Examples== | ==Examples== | ||
| Line 61: | Line 78: | ||
#DIVIDE(379.45,22) = 17.24772727272727 | #DIVIDE(379.45,22) = 17.24772727272727 | ||
#DIVIDE(-50,5) = -10 | #DIVIDE(-50,5) = -10 | ||
| + | #(10006785432188888888n).divide(2665n) = 3754891344160933 | ||
==Related Videos== | ==Related Videos== | ||
Latest revision as of 01:21, 15 April 2020
DIVIDE ()
Description
- This function shows the division of the numbers.
- Division is one of the four basic operations of arithmetic.
- Division is splitting into equal parts or groups.
- Division is the repeated subtraction and it is the opposite of Multiplication.
- The division of two natural numbers is the process of calculating the number of times one number is contained within one another.
- In DIVIDE(),first number is the dividend and second number is the divisor.
- Dividend is the number being divided.Divisor is a number that will divide the dividend exactly.
- For example,DIVIDE(20,4),20 is the dividend and 4 is the divisor.
Divisors
- An integer is called a divisor of the number if dividing by leaves no reminder.
- Divisor of a number is also called a factor of a number.
- DIVISORS(10) = 1 2 5 10
- DIVISORS(1..10)
| 1 | |||
| 1 | 2 | ||
| 1 | 3 | ||
| 1 | 2 | 4 | |
| 1 | 5 | ||
| 1 | 2 | 3 | 6 |
| 1 | 7 | ||
| 1 | 2 | 4 | 8 |
| 1 | 3 | 9 | |
| 1 | 2 | 5 | 10 |
Proper Divisor
- The proper divisors of a positive integer N are those numbers, other than N itself, that divide N without remainder.
- For N > 1 they will always include 1, but for N = 1 there are no proper divisors.
- The proper divisors of 6 are 1, 2, and 3.
- PROPERDIVISORS(10) = 1 2 5
- ALIQUOTDIVISORS(15) = 1 3 5
Examples
- DIVIDE(1000,50) = 20
- DIVIDE(300,7) = 42.857142857142854
- DIVIDE(379.45,22) = 17.24772727272727
- DIVIDE(-50,5) = -10
- (10006785432188888888n).divide(2665n) = 3754891344160933
Related Videos
See Also
References
 is called a divisor of the number
is called a divisor of the number 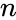 if dividing
if dividing