Difference between revisions of "Manuals/calci/GAMMADIST"
Jump to navigation
Jump to search
(Created page with "<div id="6SpaceContent" class="zcontent" align="left"> <font color="#000000">'''GAMMADIST(X, Alpha, Beta, Cum)'''</font> <br /> <font color="#000000">Where X is the...") |
|||
| (30 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | <div | + | <div style="font-size:30px">'''GAMMADIST(x,alpha,beta,cumulative,accuracy)'''</div><br/> |
| + | *<math>x</math> is the value of the distribution. | ||
| + | *<math>alpha</math> and <math>beta</math> are the value of the parameters. | ||
| + | *<math>cumulative</math> is the logical value like true or false. | ||
| + | *<math>accuracy</math> gives accurate value of the solution. | ||
| + | **GAMMADIST(), returns the gamma distribution. | ||
| − | < | + | ==Description== |
| + | *This function gives the value of the Gamma Distribution. | ||
| + | *The Gamma Distribution can be used in a queuing models like, the amount of rainfall accumulated in a reservoir. | ||
| + | *This distribution is the Continuous Probability Distribution with two parameters <math>\alpha</math> and <math>\beta</math>. | ||
| + | *In <math>GAMMADIST(x,alpha,beta,cumulative,accuracy)</math>, <math>x</math> is the value of the distribution, <math>\alpha</math> is called shape parameter and <math>\beta</math> is the rate parameter of the distribution and <math>cumulative</math> is the logical value like TRUE or FALSE. | ||
| + | *If <math>cumulative</math> is TRUE, then this function gives the Cumulative Distribution value and if is FALSE then it gives the Probability Density Function. | ||
| + | *<math>cumulative</math> gives accurate value of the solution. | ||
| + | *The gamma function is defined by : | ||
| + | <math>Gamma(t) = \int\limits_{0}^{\infty}x^{t-1} e^{-x} dx</math>. | ||
| + | *It is for all complex numbers except the negative integers and zero. | ||
| + | *The Probability Density Function of Gamma function using Shape, rate parameters is: | ||
| + | <math> f(x; \alpha,\beta)=\frac{x^{\alpha-1} e^{-\frac {x}{\beta}}}{\beta^{\alpha} \Gamma(\alpha)}</math>, for | ||
| + | :<math>x, \alpha , \beta > 0 </math>, where <math>e</math> is the natural number(e = 2.71828...), <math>\alpha</math> is the number of occurrences of an event, and <math>\Gamma(\alpha)</math> is the Gamma function. | ||
| + | *The Standard Gamma Probability Density function is: | ||
| + | <math>f(x,\alpha)=\frac{x^{\alpha-1} e^{-x}}{\Gamma(\alpha)}</math>. | ||
| + | *The Cumulative Distribution Function of Gamma is : | ||
| + | <math>F(x;\alpha,\beta)=\frac{\gamma(\alpha,\frac{x}{\beta})}{\Gamma(\alpha)}</math>, or | ||
| + | :<math>F(x;\alpha,\beta)= e^{-\frac {x}{\beta}} \sum_{i=k}^{\infty} \frac{1}{i!} (\frac{x}{\beta})^i</math> for any positive integer <math>k</math>. | ||
| + | *When alpha is a positive integer, then the distribution is called Erlang distribution. | ||
| + | *If the shape parameter <math>\alpha</math> is held fixed, the resulting one-parameter family of distributions is a natural exponential family. | ||
| + | *For a positive integer <math>n</math>, when <math>\alpha =\frac{n}{2}</math>, <math>\beta = 2</math>, and <math>cu= TRUE</math>, GAMMADIST returns (1 - CHIDIST(x)) with <math>n</math> degrees of freedom. | ||
| + | *This function shows the result as error when | ||
| + | 1.Any one of the argument is non numeric | ||
| + | 2.<math>x<0</math>, <math>\alpha \le 0</math> or <math>\beta \le 0</math>. | ||
| − | < | + | ==ZOS== |
| + | *The syntax is to calculate GAMMADIST in ZOS is <math>GAMMADIST(x,alpha,beta,cumulative,accuracy)</math>. | ||
| + | **<math>x</math> is the value of the distribution, | ||
| + | **<math>alpha</math> and <math>beta</math> are the value of the parameters | ||
| + | **<math>cumulative</math> is the logical value like true or false. | ||
| + | **<math>accuracy</math> gives accurate value of the solution. | ||
| + | *For e.g.,GAMMADIST(10.45,2.8,6.4,TRUE,0.9) | ||
| + | GAMMADIST(10.45,2.8,6.4,FALSE,0.9) | ||
| + | {{#ev:youtube|l_qRjj8bUdw|280|center|Gamma Distribution}} | ||
| − | + | ==Examples== | |
| + | #GAMMADIST(8.15372,5,7,TRUE)=0.006867292 | ||
| + | #GAMMADIST(20.78542,2,6,TRUE)=0.860283293 | ||
| + | #GAMMADIST(20.78542,2,6,FALSE)=0.01806997 | ||
| + | #GAMMADIST(45.6523,9,4,FALSE)=0.019724471 | ||
| + | #GAMMADIST(8.15372,5,7,TRUE,0.5)= 0.00693316259 | ||
| + | #GAMMADIST(8.15372,5,7,TRUE,0.9)=0.0067648564 | ||
| − | + | ==Related Videos== | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | {{#ev:youtube|SAMTXAAKeug|280|center|GAMMA Distribution}} | |
| − | * | + | ==See Also== |
| − | * | + | *[[Manuals/calci/DATE | DATE ]] |
| + | *[[Manuals/calci/DAYS360 | DAYS360]] | ||
| + | *[[Manuals/calci/DATEVALUE | DATEVALUE]] | ||
| − | + | ==References== | |
| + | [http://en.wikipedia.org/wiki/Gamma_distribution Gamma Distribution] | ||
| − | |||
| − | |||
| − | [[ | + | *[[Z_API_Functions | List of Main Z Functions]] |
| − | + | *[[ Z3 | Z3 home ]] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Latest revision as of 17:08, 7 August 2018
GAMMADIST(x,alpha,beta,cumulative,accuracy)
- is the value of the distribution.
- and are the value of the parameters.
- is the logical value like true or false.
- gives accurate value of the solution.
- GAMMADIST(), returns the gamma distribution.
Description
- This function gives the value of the Gamma Distribution.
- The Gamma Distribution can be used in a queuing models like, the amount of rainfall accumulated in a reservoir.
- This distribution is the Continuous Probability Distribution with two parameters and .
- In , is the value of the distribution, is called shape parameter and is the rate parameter of the distribution and is the logical value like TRUE or FALSE.
- If is TRUE, then this function gives the Cumulative Distribution value and if is FALSE then it gives the Probability Density Function.
- gives accurate value of the solution.
- The gamma function is defined by :
.
- It is for all complex numbers except the negative integers and zero.
- The Probability Density Function of Gamma function using Shape, rate parameters is:
, for
- , where is the natural number(e = 2.71828...), is the number of occurrences of an event, and is the Gamma function.
- The Standard Gamma Probability Density function is:
.
- The Cumulative Distribution Function of Gamma is :
, or
- for any positive integer .
- When alpha is a positive integer, then the distribution is called Erlang distribution.
- If the shape parameter is held fixed, the resulting one-parameter family of distributions is a natural exponential family.
- For a positive integer , when , , and , GAMMADIST returns (1 - CHIDIST(x)) with degrees of freedom.
- This function shows the result as error when
1.Any one of the argument is non numeric 2., or .
ZOS
- The syntax is to calculate GAMMADIST in ZOS is .
- is the value of the distribution,
- and are the value of the parameters
- is the logical value like true or false.
- gives accurate value of the solution.
- For e.g.,GAMMADIST(10.45,2.8,6.4,TRUE,0.9)
GAMMADIST(10.45,2.8,6.4,FALSE,0.9)
Examples
- GAMMADIST(8.15372,5,7,TRUE)=0.006867292
- GAMMADIST(20.78542,2,6,TRUE)=0.860283293
- GAMMADIST(20.78542,2,6,FALSE)=0.01806997
- GAMMADIST(45.6523,9,4,FALSE)=0.019724471
- GAMMADIST(8.15372,5,7,TRUE,0.5)= 0.00693316259
- GAMMADIST(8.15372,5,7,TRUE,0.9)=0.0067648564
Related Videos
See Also
References
 is the value of the distribution.
is the value of the distribution. and
and  are the value of the parameters.
are the value of the parameters.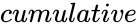 is the logical value like true or false.
is the logical value like true or false.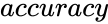 gives accurate value of the solution.
gives accurate value of the solution.
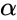 and
and  .
.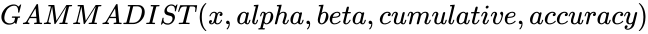 ,
, 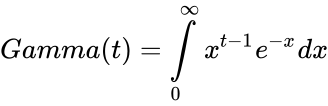 .
.
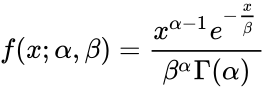 , for
, for
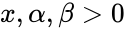 , where
, where 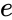 is the natural number(e = 2.71828...),
is the natural number(e = 2.71828...), 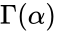 is the Gamma function.
is the Gamma function.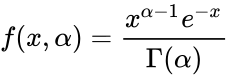 .
.
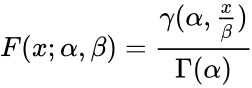 , or
, or
 for any positive integer
for any positive integer 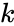 .
.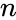 , when
, when 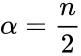 ,
, 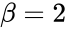 , and
, and 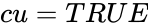 , GAMMADIST returns (1 - CHIDIST(x)) with
, GAMMADIST returns (1 - CHIDIST(x)) with 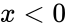 ,
,  or
or 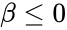 .
.