Difference between revisions of "Manuals/calci/MDETERM"
Jump to navigation
Jump to search
| Line 1: | Line 1: | ||
| − | <div style="font-size:30px">'''MDETERM( | + | <div style="font-size:30px">'''MDETERM(a)'''</div><br/> |
| − | *<math> | + | *<math>a</math> is the array of numeric elements |
| + | |||
==Description== | ==Description== | ||
*This function gives the determinant value of a matrix. | *This function gives the determinant value of a matrix. | ||
| Line 39: | Line 40: | ||
1. Any one of the element in array is empty or contain non-numeric | 1. Any one of the element in array is empty or contain non-numeric | ||
2. Number of rows is not equal to number of columns | 2. Number of rows is not equal to number of columns | ||
| + | |||
| + | ==ZOS Section== | ||
| + | *The syntax is to calculate determinant of a matrix in ZOS is <math>MDETERM(a)</math>. | ||
| + | **<math>a</math> is the array of numeric elements. | ||
| + | *For e.g., | ||
==Examples== | ==Examples== | ||
Revision as of 23:34, 2 July 2014
MDETERM(a)
- is the array of numeric elements
Description
- This function gives the determinant value of a matrix.
- To calculate the determinant of a matrix, we can choose only square matrix.i.e. Number of rows and number of columns should be equal.
- Determinant of the identity matrix is always 1.
- Determinant of the matrix is denoted by or .
- Let be 2x2 matrix with the elements
- Then , where all are real numbers.
- Let be the 3x3 matrix with the elements
Then :
- Let be a square matrix of order . Write ,
- Where is the entry on the row and column and to & to .
- For any and , set (called the co-factors), then the general formula for determinant of the matrix is,
, for any fixed . Also, for any fixed .
- This function will give the result as error when
1. Any one of the element in array is empty or contain non-numeric 2. Number of rows is not equal to number of columns
ZOS Section
- The syntax is to calculate determinant of a matrix in ZOS is .
- is the array of numeric elements.
- For e.g.,
Examples
- =MDETERM({6,4,8;3,6,1;2,4,5}) = 104
- =MDETERM({-5,10;6,-8}) = -20
- =MDETERM({1,0,2,1;4,0,2,-1;1,4,5,2;3,1,2,0}) = 17
- =MDETERM({1,2,3;5,2,8}) = NAN
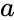 is the array of numeric elements
is the array of numeric elements is denoted by
is denoted by 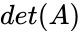 or
or 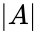 .
.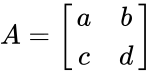
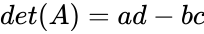 , where
, where  all are real numbers.
all are real numbers.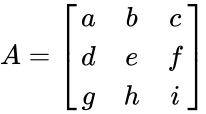 Then
Then 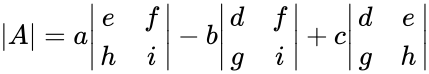 :
:

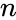 . Write
. Write 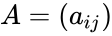 ,
,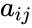 is the entry on the
is the entry on the 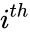 row and
row and 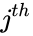 column and
column and 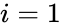 to
to 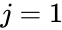 to
to 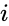 and
and 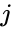 , set
, set 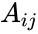 (called the co-factors), then the general formula for determinant of the matrix
(called the co-factors), then the general formula for determinant of the matrix  , for any fixed
, for any fixed 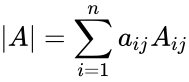 , for any fixed
, for any fixed 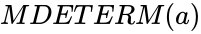 .
.