Difference between revisions of "Manuals/calci/HANKEL"
Jump to navigation
Jump to search
| Line 32: | Line 32: | ||
| 0.6076015164144337 || 0.6414852568414062 || 0.9679132911842316 | | 0.6076015164144337 || 0.6414852568414062 || 0.9679132911842316 | ||
|} | |} | ||
| + | #MATRIX("hankel",5,1..10) | ||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | 1 ||2 || 3 || 4 ||5 | ||
| + | |- | ||
| + | | 2 || 3 || 4 || 5 || 6 | ||
| + | |- | ||
| + | | 3 || 4 || 5 || 6 || 7 | ||
| + | |- | ||
| + | | 4 || 5 || 6 || 7 || 8 | ||
| + | |- | ||
| + | | 5 || 6 || 7 || 8 ||9 | ||
| + | |} | ||
| + | #MATRIX("hankel",5,-10..0) | ||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | -10 ||-9 || -8 || -7 || -6 | ||
| + | |- | ||
| + | | -9 || -8 || -7 || -6 || -5 | ||
| + | |- | ||
| + | | -8 || -7 || -6 || -5 || -4 | ||
| + | |- | ||
| + | | -7 || -6 || -5 || -4 || -3 | ||
| + | |- | ||
| + | | -6 || -5 || -4 || -3 || -2 | ||
| + | |} | ||
| + | #MATRIX("hankel",4,["rice","water"]) | ||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | rice || water || rice || water | ||
| + | |- | ||
| + | | water || rice || water || rice | ||
| + | |- | ||
| + | | rice || water || rice || water || | ||
| + | |- | ||
| + | | water || rice || water || rice | ||
| + | |} | ||
| + | |||
| + | ==See Also== | ||
| + | *[[Manuals/calci/ANTIDIAGONAL| ANTIDIAGONAL]] | ||
| + | *[[Manuals/calci/CONFERENCE| CONFERENCE]] | ||
| + | *[[Manuals/calci/CIRCULANT| CIRCULANT]] | ||
| + | *[[Manuals/calci/HADAMARD| HADAMARD]] | ||
| + | |||
| + | ==References== | ||
Revision as of 10:53, 24 April 2015
HANKEL
MATRIX("HANKEL",order)
- is the order of the hankel matrix.
Description
- This function gives the matrix with the property of hankel matrix.
- A hankel matrix is a square matrix with constant skew diagonals.
- If the i,j element of Hankel matrix A is denoted , then we have
.
- i.e., The form of Hankel matrix is:
.
- A hankel matrix is also called as catalecticant matrix.
- Here MATRIX("hankel") is gives the hankel matrix of order 3 with decimal values.
- A Hankel matrix is an upside-down Toeplitz matrix.
- A matrix whose entries along a parallel to the main anti-diagonal are equal, for each parallel.
- Equivalently, is a Hankel matrix if and only if there exists a sequence, such that ,If are square matrices, then is referred to as a block Hankel matrix.
Examples
- MATRIX("hankel")
| 0.6414852568414062 | 0.9679132911842316 | 0.6076015164144337 |
| 0.9679132911842316 | 0.6076015164144337 | 0.6414852568414062 |
| 0.6076015164144337 | 0.6414852568414062 | 0.9679132911842316 |
- MATRIX("hankel",5,1..10)
| 1 | 2 | 3 | 4 | 5 |
| 2 | 3 | 4 | 5 | 6 |
| 3 | 4 | 5 | 6 | 7 |
| 4 | 5 | 6 | 7 | 8 |
| 5 | 6 | 7 | 8 | 9 |
- MATRIX("hankel",5,-10..0)
| -10 | -9 | -8 | -7 | -6 |
| -9 | -8 | -7 | -6 | -5 |
| -8 | -7 | -6 | -5 | -4 |
| -7 | -6 | -5 | -4 | -3 |
| -6 | -5 | -4 | -3 | -2 |
- MATRIX("hankel",4,["rice","water"])
| rice | water | rice | water | |
| water | rice | water | rice | |
| rice | water | rice | water | |
| water | rice | water | rice |
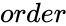 is the order of the hankel matrix.
is the order of the hankel matrix.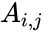 , then we have
, then we have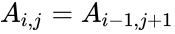 .
.
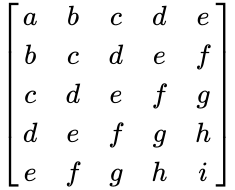 .
.