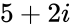Difference between revisions of "Manuals/calci/CHIDIST"
Jump to navigation
Jump to search
(Created page with "<div id="6SpaceContent" class="zcontent" align="left"> <font size="3"><font face="Times New Roman">'''CHIDIST''' ('''n''',''' df''')</font></font> <font size="3"><font f...") |
|||
| Line 1: | Line 1: | ||
| − | <div | + | <div style="font-size:30px">'''CHIDIST(x,df)'''</div><br/> |
| − | + | *'X' is the value for which distribution is evaluated and df is the number of degrees of freedem. | |
| − | + | ==Description== | |
| + | *This function gives the one_tailed probability of the chi-squared distribution. | ||
| + | *It is denoted by X^2 distribution.Normally categorical datas may displayed in tables. | ||
| + | *The X^2 static used to compare the observed value in each table to the value | ||
| + | *which would be the expected under the assumption. The conditions of X^2 test is | ||
| − | + | 1.The table should be 2x2 or more than 2x2 | |
| − | -- | + | 2.Each observations should not be dependent |
| − | < | + | 3.All expected values should be 10 or greater. |
| + | The test statistic is: | ||
| + | X^2=summation(Oi-Ei)^2/Ei | ||
| + | The degrees of freedom are: (r–1)(c–1) | ||
| + | r =No. of rows and c = No. of columns | ||
| + | Where: | ||
| + | Oi-the observed value in the ith cell | ||
| + | Ei- the expected value in the ith cell | ||
| + | Also this function will the result as Error when | ||
| + | 1.The x&df values are nonnumeric | ||
| + | 2.The x value is negative or df value is not an integer | ||
| + | 3. The df <1or df>10^10 | ||
| + | 4.Here CHIDIST=P(X>x),where X is a X^2 random variable. | ||
| − | < | + | **<math>=COMPLEX(5,2)</math> gives <math>5+2i</math> |
| + | **<math>=COMPLEX(5,2,"j")</math> gives <math>5+2j</math> | ||
| − | + | ==Examples== | |
| − | + | {| id="TABLE3" class="SpreadSheet blue" | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | {| id=" | ||
|- class="even" | |- class="even" | ||
| − | | | + | | Complex(RN,IN,SF) |
| − | + | ! RN | |
| − | + | ! IN | |
| − | + | ! SF | |
| − | + | ! RESULT | |
| − | + | |- | |
| − | | | ||
|- class="odd" | |- class="odd" | ||
| − | | | + | |Complex(5,6) |
| − | | | + | |5 |
| − | + | |6 | |
| − | |||
| − | |||
| − | |||
| | | | ||
| + | |5+6i | ||
|- class="even" | |- class="even" | ||
| − | | | + | |Complex(5,2,"j") |
| − | | | + | |5 |
| − | | | + | |2 |
| − | | | + | |j |
| − | + | |5+6j | |
| − | |||
| − | |||
| − | | | ||
|- class="odd" | |- class="odd" | ||
| − | | | + | | Complex(2,0,"i") |
| − | + | |2 | |
| − | | | + | |0 |
| − | | | + | |i |
| − | | | + | |2 |
| − | |||
| − | | | ||
|- class="even" | |- class="even" | ||
| − | | | + | | Complex(0,-4,i) |
| − | + | |0 | |
| − | + | |4 | |
| − | | | + | |i |
| − | | | + | |4i |
| − | | | ||
| − | | | ||
|- class="odd" | |- class="odd" | ||
| − | | | + | |Complex(5,"j") |
| − | + | |5 | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | | | ||
| | | | ||
| + | |j | ||
| + | |Error | ||
|} | |} | ||
| − | + | ==See Also== | |
| − | + | ||
| + | *[[Manuals/calci/IMAGINARY | IMAGINARY]] | ||
| + | *[[Manuals/calci/IMREAL| IMREAL]] | ||
| + | |||
| + | ==References== | ||
| + | [http://en.wikipedia.org/wiki/Complex_number| Complex Numbers] | ||
Revision as of 05:02, 12 November 2013
CHIDIST(x,df)
- 'X' is the value for which distribution is evaluated and df is the number of degrees of freedem.
Description
- This function gives the one_tailed probability of the chi-squared distribution.
- It is denoted by X^2 distribution.Normally categorical datas may displayed in tables.
- The X^2 static used to compare the observed value in each table to the value
- which would be the expected under the assumption. The conditions of X^2 test is
1.The table should be 2x2 or more than 2x2 2.Each observations should not be dependent 3.All expected values should be 10 or greater. The test statistic is: X^2=summation(Oi-Ei)^2/Ei The degrees of freedom are: (r–1)(c–1) r =No. of rows and c = No. of columns Where: Oi-the observed value in the ith cell Ei- the expected value in the ith cell Also this function will the result as Error when 1.The x&df values are nonnumeric 2.The x value is negative or df value is not an integer 3. The df <1or df>10^10 4.Here CHIDIST=P(X>x),where X is a X^2 random variable.
- gives
- Failed to parse (syntax error): {\displaystyle =COMPLEX(5,2,"j")} gives
Examples
| Complex(RN,IN,SF) | RN | IN | SF | RESULT |
|---|---|---|---|---|
| Complex(5,6) | 5 | 6 | 5+6i | |
| Complex(5,2,"j") | 5 | 2 | j | 5+6j |
| Complex(2,0,"i") | 2 | 0 | i | 2 |
| Complex(0,-4,i) | 0 | 4 | i | 4i |
| Complex(5,"j") | 5 | j | Error |
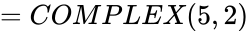 gives
gives