Difference between revisions of "Manuals/calci/HARMEAN"
Jump to navigation
Jump to search
(Created page with "<div id="6SpaceContent" class="zcontent" align="left"> <font size="3"><font face="Times New Roman">'''HARMEAN''' (N'''1''', N2...)</font></font> <font size="3"><font fac...") |
|||
| Line 1: | Line 1: | ||
| − | <div | + | <div style="font-size:30px">'''HARMEAN(n1,n2)'''</div><br/> |
| + | *<math>n1</math> and <math>n2 </math> are the positive real numbers. | ||
| + | ==Description== | ||
| + | *This function gives the harmonic mean of a given set of numbers. | ||
| + | *Harmonic mean is used to calculate the average of a set of numbers. | ||
| + | *The Harmonic mean is always the lowest mean. | ||
| + | *Normally Harmonic mean<geometric mean<Arithmetic mean. | ||
| + | *Harmonic mean is dfined by the reciprocal of the arithmetic mean of the reciprocals of a specified set of numbers. | ||
| + | *The harmonic mean of a positive real numbers x1,x2,x3....xn>0 is defined by H=n/(1/x1+1/x2+...+1/xn)=n/summation (i=1 to n)(1/xi). | ||
| + | *In HARMEAN(n1,n2,...) n1,n2.. are the positive real numbers, and here n1 is required.n2,n3..., are optional. | ||
| + | *Also arguments can be numbers,names, arrays or references that contain numbers. | ||
| + | *We can give logical values and text representations of numbers directly. Suppose the arguments contains any text, logical values or empty cells like that values are ignored. | ||
| + | *This will give the result as error when 1.the arguments with the error values or the referred text couldn't translated in to numbers. | ||
| + | 2.Also any data point<=0. | ||
| + | ==Examples== | ||
| − | + | HARMEAN(1,2,3,4,5)=2.18978102189781 | |
| + | HARMEAN(20,25,32,41)=27.4649361523969 | ||
| + | HARMEAN(0.25,5.4,3.7,10.1,15.2)=1.0821913906985883 | ||
| + | HARMEAN(3,5,0,2)=NAN | ||
| + | HARMEAN(1,-2,4)=NAN | ||
| − | + | ==See Also== | |
| + | *[[Manuals/calci/AVERAGE | AVERAGE ]] | ||
| + | *[[Manuals/calci/GEOMEAN | GEOMEAN ]] | ||
| − | |||
| − | |||
| − | |||
| − | + | ==References== | |
| − | + | [http://en.wikipedia.org/wiki/Pearson_product-moment_correlation_coefficient| Correlation] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | - | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Revision as of 01:10, 10 December 2013
HARMEAN(n1,n2)
- and are the positive real numbers.
Description
- This function gives the harmonic mean of a given set of numbers.
- Harmonic mean is used to calculate the average of a set of numbers.
- The Harmonic mean is always the lowest mean.
- Normally Harmonic mean<geometric mean<Arithmetic mean.
- Harmonic mean is dfined by the reciprocal of the arithmetic mean of the reciprocals of a specified set of numbers.
- The harmonic mean of a positive real numbers x1,x2,x3....xn>0 is defined by H=n/(1/x1+1/x2+...+1/xn)=n/summation (i=1 to n)(1/xi).
- In HARMEAN(n1,n2,...) n1,n2.. are the positive real numbers, and here n1 is required.n2,n3..., are optional.
- Also arguments can be numbers,names, arrays or references that contain numbers.
- We can give logical values and text representations of numbers directly. Suppose the arguments contains any text, logical values or empty cells like that values are ignored.
- This will give the result as error when 1.the arguments with the error values or the referred text couldn't translated in to numbers.
2.Also any data point<=0.
Examples
HARMEAN(1,2,3,4,5)=2.18978102189781 HARMEAN(20,25,32,41)=27.4649361523969 HARMEAN(0.25,5.4,3.7,10.1,15.2)=1.0821913906985883 HARMEAN(3,5,0,2)=NAN HARMEAN(1,-2,4)=NAN
See Also
 and
and 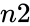 are the positive real numbers.
are the positive real numbers.