Difference between revisions of "Manuals/calci/IMARGUMENT"
Jump to navigation
Jump to search
(Created page with "<div id="6SpaceContent" class="zcontent" align="left"> <font color="#000000"><font face="Arial, sans-serif"><font size="2">'''IMARGUMENT'''</font></font><font face="Arial, sa...") |
|||
| Line 1: | Line 1: | ||
| − | <div | + | <div style="font-size:30px">'''IMARGUMENT(z)'''</div><br/> |
| + | *<math>z</math> is the complex number is of the form <math>x+iy</math> | ||
| + | *<math>n</math> is the order of the Bessel function and is an integer | ||
| + | ==Description== | ||
| + | *This function gives the principal value of the argument of the complex-valued expression z. i.e ., | ||
| + | *The angle from the positive axis to the line segment is called the argument of a complex number. | ||
| + | *In this function angle value is in radians. | ||
| + | *Here IMARGUMENT(z), Where z is the complex number in the form of "x+iy".i.e. x&y are the real numbers. | ||
| + | *'I' imaginary unit .i=sqrt(-1). | ||
| + | *An argument of the complex number z = x + iy is any real quantity φ such that z = x + i y = r cosφ + i r sinφ for some positive real number r. | ||
| + | *Where r=|z|=sqrt(x^2+y^2) and φ∈(is belongs to) (-Pi(),Pi()]. | ||
| + | *The argument of a complex number is calculated by arg(z)= tan^-1(y/x) =theta in radians. | ||
| + | *To change the radian value in to degree we can use DEGREES function or we can multiply the answer with 180/pi(). | ||
| + | *We can use COMPLEX function to convert real and imaginary number in to a complex number. | ||
| − | + | ==Examples== | |
| − | + | #IMARGUMENT("3-2i")=-0.588002604 | |
| − | + | #IMARGUMENT("5+6i")=0.876058051 | |
| − | + | #IMARGUMENT("2")=0 | |
| + | #IMARGUMENT("4i")=1.570796327 | ||
| + | #DEGREES(IMARGUMENT("2+2i"))=45 | ||
| − | |||
| − | |||
| − | + | ==References== | |
| − | + | [http://en.wikipedia.org/wiki/Bessel_function Bessel Function] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Revision as of 22:56, 15 December 2013
IMARGUMENT(z)
- is the complex number is of the form
- is the order of the Bessel function and is an integer
Description
- This function gives the principal value of the argument of the complex-valued expression z. i.e .,
- The angle from the positive axis to the line segment is called the argument of a complex number.
- In this function angle value is in radians.
- Here IMARGUMENT(z), Where z is the complex number in the form of "x+iy".i.e. x&y are the real numbers.
- 'I' imaginary unit .i=sqrt(-1).
- An argument of the complex number z = x + iy is any real quantity φ such that z = x + i y = r cosφ + i r sinφ for some positive real number r.
- Where r=|z|=sqrt(x^2+y^2) and φ∈(is belongs to) (-Pi(),Pi()].
- The argument of a complex number is calculated by arg(z)= tan^-1(y/x) =theta in radians.
- To change the radian value in to degree we can use DEGREES function or we can multiply the answer with 180/pi().
- We can use COMPLEX function to convert real and imaginary number in to a complex number.
Examples
- IMARGUMENT("3-2i")=-0.588002604
- IMARGUMENT("5+6i")=0.876058051
- IMARGUMENT("2")=0
- IMARGUMENT("4i")=1.570796327
- DEGREES(IMARGUMENT("2+2i"))=45
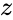 is the complex number is of the form
is the complex number is of the form 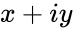
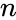 is the order of the Bessel function and is an integer
is the order of the Bessel function and is an integer