Difference between revisions of "Manuals/calci/TDIST"
Jump to navigation
Jump to search
| Line 15: | Line 15: | ||
*Suppose t=1, then this distribution is one-tailed distribution and t=2, then this is two-tailed distribution. | *Suppose t=1, then this distribution is one-tailed distribution and t=2, then this is two-tailed distribution. | ||
*Also t=1, then it is calculated as <math> TDIST=P(X>x) </math>, where <math> X </math> is a random variable that follows the t-distribution. | *Also t=1, then it is calculated as <math> TDIST=P(X>x) </math>, where <math> X </math> is a random variable that follows the t-distribution. | ||
| − | *And t=2, then it is calculated as <math> TDIST =P(X>x or X<-x)</math>. | + | *And t=2, then it is calculated as <math> TDIST =P(X>x or X<-x) </math>. |
*This function will return the result as error | *This function will return the result as error | ||
1. Any one of the argument is nonnumeric. | 1. Any one of the argument is nonnumeric. | ||
2. df<1 and x<0. When we are giving df and t as a decimals, then it is changing in to integers. | 2. df<1 and x<0. When we are giving df and t as a decimals, then it is changing in to integers. | ||
| − | |||
==Examples== | ==Examples== | ||
Revision as of 23:35, 26 January 2014
TDIST(x,df,t),
- is the numeric value to find the distribution.
- is the degrees of freedom.
- is the number of tails.
Description
- This function gives the value of the t-distribution.
- It is the continuous probability distributions.
- The t-distribution is also called students t-distribution.
- This is the symmetric distribution like the normal distribution.
- It is used when making inferences about a population mean when the population standard deviation is not known.
- In is the numeric value to find the value of the distribution.
- is the integer which is indicating the number of degrees of freedom and is indicating the number of distribution tails.
- Suppose t=1, then this distribution is one-tailed distribution and t=2, then this is two-tailed distribution.
- Also t=1, then it is calculated as , where is a random variable that follows the t-distribution.
- And t=2, then it is calculated as .
- This function will return the result as error
1. Any one of the argument is nonnumeric.
2. df<1 and x<0. When we are giving df and t as a decimals, then it is changing in to integers.
Examples
- TDIST(1.82,55,1) = 0.037101192599
- TDIST(1.82,55,2) = 0.074202385199
- TDIST(5.9812,75,1)= 3.50350792266e-8
- TDIST(5.9812,75,2) = 7.007015845328e-8
- TDIST(2.4579,20.4,1) = 0.0122238
- TDIST(2.4579,20.4,1.2) = Null
See Also
 is the numeric value to find the distribution.
is the numeric value to find the distribution.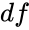 is the degrees of freedom.
is the degrees of freedom.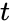 is the number of tails.
is the number of tails. is the numeric value to find the value of the distribution.
is the numeric value to find the value of the distribution.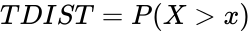 , where
, where 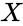 is a random variable that follows the t-distribution.
is a random variable that follows the t-distribution.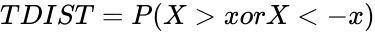 .
.