Difference between revisions of "Manuals/calci/CHOLESKY"
Jump to navigation
Jump to search
(Created page with "<div style="font-size:30px">'''CHOLESKY'''</div><br/>") |
|||
| Line 1: | Line 1: | ||
| − | <div style="font-size:30px">'''CHOLESKY'''</div><br/> | + | <div style="font-size:30px">'''CHOLESKY(ar1)'''</div><br/> |
| + | *<math>a</math> is the array of numeric elements | ||
| + | |||
| + | ==Description== | ||
| + | *This function gives the value of Cholesky factorization. | ||
| + | *It is called cholesky decomposition or cholesky factorization. | ||
| + | *The Cholesky factorization is only defined for symmetric or Hermitian positive definite matrices.Every positive definite matrix A can be factored as <math>A</math> = <math>LL^{T}</math> | ||
| + | where: | ||
| + | <math>L</math> is lower triangular with positive diagonal elements | ||
| + | <math>L^{T}</math> is is the conjugate transpose value of <math>L</math>. | ||
| + | *Every Hermitian positive-definite matrix (and thus also every real-valued symmetric positive-definite matrix) has a unique Cholesky decomposition. | ||
| + | *Here <math>CHOLESKY(array)</math>,array is set of values to find the factorization value. | ||
| + | Partition matrices in as | ||
| + | A= LL^T (Please take the description from http://www.seas.ucla.edu/~vandenbe/103/lectures/chol.pdf ) | ||
Revision as of 05:18, 8 April 2015
CHOLESKY(ar1)
- is the array of numeric elements
Description
- This function gives the value of Cholesky factorization.
- It is called cholesky decomposition or cholesky factorization.
- The Cholesky factorization is only defined for symmetric or Hermitian positive definite matrices.Every positive definite matrix A can be factored as =
where:
is lower triangular with positive diagonal elements is is the conjugate transpose value of .
- Every Hermitian positive-definite matrix (and thus also every real-valued symmetric positive-definite matrix) has a unique Cholesky decomposition.
- Here ,array is set of values to find the factorization value.
Partition matrices in as A= LL^T (Please take the description from http://www.seas.ucla.edu/~vandenbe/103/lectures/chol.pdf )
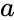 is the array of numeric elements
is the array of numeric elements =
= 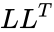
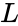 is lower triangular with positive diagonal elements
is lower triangular with positive diagonal elements
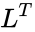 is is the conjugate transpose value of
is is the conjugate transpose value of 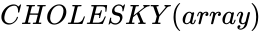 ,array is set of values to find the factorization value.
,array is set of values to find the factorization value.