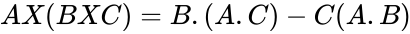Difference between revisions of "Manuals/calci/DYADIC"
Jump to navigation
Jump to search
| Line 20: | Line 20: | ||
\end{pmatrix}</math> | \end{pmatrix}</math> | ||
*The direct product is non-commutative <math>(AB \neq BA)</math>. | *The direct product is non-commutative <math>(AB \neq BA)</math>. | ||
| − | *A few vector product identities are of interest | + | *A few vector product identities are of interest: |
<math>A.BXC = AXB.C=B.CXA=BXC.A=C.AXB</math> | <math>A.BXC = AXB.C=B.CXA=BXC.A=C.AXB</math> | ||
<math>AX(BXC)= B.(A.C)- C(A.B)</math> | <math>AX(BXC)= B.(A.C)- C(A.B)</math> | ||
Revision as of 14:52, 3 March 2017
DYADIC(a,b)
OR VECTORDIRECTPRODUCT (a,b)
- and any two set of values.
Description
- This function shows the Vector Direct product.
- The third type of vector multiplication is called the direct product, and is written AB.
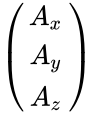
- In , and are the two vectors.
- Multiplying one vector by another under the direct product gives a tensor result.
- The rectangular components of the direct product may be found by matrix multiplication: one multiplies the column vector A by the transpose of B, which gives a 3X3 matrix:
= =
- The direct product is non-commutative .
- A few vector product identities are of interest:
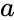 and
and 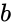 any two set of values.
any two set of values.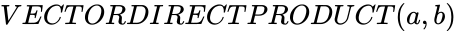 ,
, 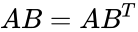 =
=
 =
=
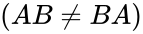 .
.