Difference between revisions of "Manuals/calci/LB"
Jump to navigation
Jump to search
(Created page with "LB") |
|||
| Line 1: | Line 1: | ||
| − | LB | + | <div style="font-size:30px">'''LB (Number)'''</div><br/> |
| + | *<math>Number</math> is any positive real number. | ||
| + | |||
| + | ==Description== | ||
| + | *This function shows the logarithm value of base 2. | ||
| + | *In <math>LB(Number)</math>,<math>Number</math> is any positive real number. | ||
| + | *LB means the logarithm with the base 2. | ||
| + | *So the Binary logarithm is the logarithm to the base 2. | ||
| + | *The Binary logarithm function is the inverse function of the power of two function. | ||
| + | *So for any real number x, <math>x= log_{2} n</math> ,<math>2^x=n</math>. | ||
| + | *For example, the binary logarithm of 1 is 0, the binary logarithm of 2 is 1, the binary logarithm of 4 is 2, and the binary logarithm of 32 is 5. | ||
| + | *This function will return the result as NaN when the given number as negative. | ||
Revision as of 14:01, 29 March 2017
LB (Number)
- is any positive real number.
Description
- This function shows the logarithm value of base 2.
- In , is any positive real number.
- LB means the logarithm with the base 2.
- So the Binary logarithm is the logarithm to the base 2.
- The Binary logarithm function is the inverse function of the power of two function.
- So for any real number x, ,.
- For example, the binary logarithm of 1 is 0, the binary logarithm of 2 is 1, the binary logarithm of 4 is 2, and the binary logarithm of 32 is 5.
- This function will return the result as NaN when the given number as negative.
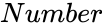 is any positive real number.
is any positive real number. ,
,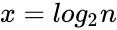 ,
, .
.