Manuals/calci/STDEV
STDEV(n1,n2,n3…)
- are numbers.
Description
- This function gives the Standard Deviation based on a given sample.
- Standard Deviation is the quantity expressed by, how many members of a group differ from the mean value of the group.
- It is the used as a measure of the dispersion or variation in a distribution.
- It is calculated as the square root of variance.
- In , , are numbers to find the Standard Deviation.
- Here is required. are optional.
- Instead of numbers, we can use the single array or a reference of a array.
- is defined by the formula:
where is the sample mean of and is the total numbers of the given data.
- It is calculated using Failed to parse (syntax error): {\displaystyle "n-1"} method.
- This function is considering our given data is the sample of the population.
- Suppose it should consider the data as the entire population, we can use the STDEVP function.
- The arguments can be be either numbers or names, array,constants or references that contain numbers.
- Suppose the array contains text,logical values or empty cells, like that values are not considered.
- When we are entering logical values and text representations of numbers as directly, then the arguments are counted.
- Suppose the function have to consider the logical values and text representations of numbers in a reference , we can use the STDEVA function.
- This function will return the result as error when
1. Any one of the argument is non-numeric.
2. The arguments containing the error values or text that cannot be translated in to numbers.
Examples
| A | B | C | D | E | F | |
|---|---|---|---|---|---|---|
| 1 | 0 | 4 | 6 | 10 | 12 | 15 |
| 2 | 7 | 3 | -1 | 2 | 25 | |
| 3 | 9 | 11 | 8 | 6 | 15 |
- =STDEV(18,25,76,91,107) = 39.8660256358
- =STDEV(208,428,511,634,116,589,907) = 267.0566196431
- =STDEV(A1:F1) = 5.52871293039
- =STDEV(A2:D2) = 3.304037933599
- =STDEV(A3:B3) = 1.414213562373
See Also
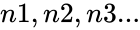 are numbers.
are numbers. ,
,  is required.
is required. 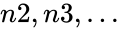 are optional.
are optional. is defined by the formula:
is defined by the formula: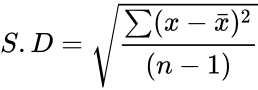
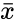 is the sample mean of
is the sample mean of  and
and 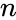 is the total numbers of the given data.
is the total numbers of the given data.