Manuals/calci/CORREL
CORREL(ar1,ar2)
- and are the set of values.
Description
- This function gives the correlation coefficient of the 1st set( ) of values and 2nd set( ) of values.
- Correlation is a statistical technique which shows the relation of strongly paired variables.
- For example, test average and study time are related; those who spending more time to study will get high marks and Average will go down for those who spend less time for studies.
- There are different correlation techniques to measure the Degree of Correlation.
- The most common of these is the Pearson Correlation Coefficient denoted by r xy.
- The main result of a correlation is called the Correlation Coefficient( )which ranges from -1 to +1.
- The value is positive i.e +1 when the two set values increase together then it is the perfect Positive Correlation.
- The value is negative i.e. (-1) when one value decreases as the other increases then it is called Negative Correlation.
- Suppose the value is 0 then there is no correlation (the values don't seem linked at all).
- If we have a series of measurements of and written as and where = 1, 2,...n then the Sample Correlation Coefficient is:
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle CORREL(X,Y)= r_{xy}=\frac{\sum_{i=1}^n(xi-\bar x)(yi-\bar y)}{\sqrt{ \sum_{i=1}^n (xi-\bar x)^2 \sum{i=1}^n(yi-\bar y)^2}}
, where x(bar) and y(bar) are the sample means of X and Y. *This function will give the result as error when
- ar1 and ar2 are nonnumeric or different number of data points.
- ar1 or ar2 is empty
- The denominator value is zero.
- Suppose ar1 and ar2 contains any text, logical values, or empty cells, like that values are ignored.
Examples
- 1. Find the correlation coefficients for X and Y values are given below :X={1,2,3,4,5}; Y={11,22,34,43,56}
CORREL(A4:A8,B4:B8)=0.99890610723867
- The following table gives the math scores and times taken to run 100 m for 10 friends:SCORE(X)={52,25,35,90,76,40}; TIME TAKEN(Y)={11.3,12.9,11.9,10.2,11.1,12.5}CORREL(A5:A10,B5:B10)= -0.93626409417769
- Find the correlation coefficients for X and Y values are given below :X={-4,11,34,87};Y={9,2,59,24} CORREL(A1:A4,B1:B4)=0.353184665607273
See Also
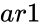 and
and 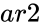 are the set of values.
are the set of values.