Manuals/calci/ZTESTEQUALMEANS
ZTESTTWOSAMPLEFORMEANS(ar1,ar2,v1,v2,md,alpha,lv)
- and are array of values.
- and are value of variances.
- is the Hypothesized Mean Difference.
- is the significance level.
- is the logical value.
Description
- This function gives the z-test two sample for means.
- We can use this test when
1.The samples can be different sizes. 2.The two samples are independent. 3.Both populations are normally distributed or both sample sizes are large enough that the means are normally distributed.
- In , and are the set of values, is the variance of , is the variance of .
- is the Hypothesized Mean Difference. If testing is for equal means,then .
- is the significance level which ranges from 0 to 1.
- is the logical value like TRUE or FALSE.TRUE is indicating the result will display in new worksheet.
- Suppose we are omitted the value it will consider the value as FALSE.
- ZTEST two sample for means is calculated by:
where and are average of two samples, is the Hypothesized Mean Difference between two means of the population. and are the standard deviation of two population. and are the sizes of the samples.
- This function will give the result as error when
1. any one of the argument is nonnumeric. 2. alpha>1
Examples
- Example 1
| A | B | |
|---|---|---|
| 1 | 70.25 | 90.02 |
| 2 | 82.87 | 89.45 |
| 3 | 90.17 | 70.89 |
| 4 | 45.55 | 107.91 |
| 5 | 51.98 | 59.09 |
| 6 | 21.28 | 45.03 |
| 7 | 39.04 | 56.08 |
| 8 | 40.47 | 91 |
| 9 | 30.02 | 40.41 |
| 10 | 100.05 | 29.04 |
| 11 | 86.1 | 37.79 |
| 12 | 72.49 | 41.07 |
| 13 | 29.54 | 52.04 |
| 14 | 38.76 | 60.78 |
| 15 | 47.01 | 66.06 |
| 16 | 50.65 | 78.01 |
| 17 | 55.91 | 41.22 |
| 18 | 102.7 | 33.99 |
| 19 | 10 | 110.02 |
- =ZTESTTWOSAMPLEFORMEANS(A1:A18,B1:B19,641.8474654,630.283176,0,0.5)
See Also
- This function gives the probability of the uniform distribution.
- Uniform distribution is a symmetric probability distribution.
- It is also called rectangular distribution.
- In , is the numeric value to find the probability of the distribution, is the lower limit value and is the upper limit value.
- The probability density function of the uniform distribution in the interval [a,b] are :
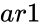 and
and 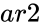 are array of values.
are array of values. and
and  are value of variances.
are value of variances.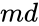 is the Hypothesized Mean Difference.
is the Hypothesized Mean Difference. is the significance level.
is the significance level. is the logical value.
is the logical value.