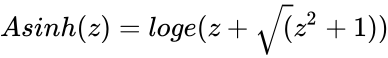Difference between revisions of "Manuals/calci/ASINH"
Jump to navigation
Jump to search
| Line 5: | Line 5: | ||
*This function gives the Inverse Hyperbolic Sine of a number. | *This function gives the Inverse Hyperbolic Sine of a number. | ||
*Here 'z' is any real number. | *Here 'z' is any real number. | ||
| − | *Inverse Hyperbolic Sine of a number is defined | + | *Inverse Hyperbolic Sine of a number is defined as <math> Asinh(z) = loge(z +\sqrt(z^2 + 1))</math> |
*ASINH(-z) = -ASINH(z) | *ASINH(-z) = -ASINH(z) | ||
Revision as of 22:35, 5 November 2013
ASINH(z)
- where z is any real number
Description
- This function gives the Inverse Hyperbolic Sine of a number.
- Here 'z' is any real number.
- Inverse Hyperbolic Sine of a number is defined as
- ASINH(-z) = -ASINH(z)
Examples
ASINH(z)
- z is any real number.
| ASINH(z) | Value(Radian) |
| ASINH(2) | 1.44363547517881 |
| ASINH(45) | 4.499933104264103 |
| ASINH(-90) | -5.192987713658952 |