Difference between revisions of "Manuals/calci/MINVERSE"
Jump to navigation
Jump to search
| Line 22: | Line 22: | ||
</math> | </math> | ||
*Now let <math>A</math> be the matrix is of order <math>nXn</math>. | *Now let <math>A</math> be the matrix is of order <math>nXn</math>. | ||
| − | *Then the inverse of <math>A</math> is <math>A^{-1}= \frac{1}{det(A)}*adj(A)<math> | + | *Then the inverse of <math>A</math> is <math>A^{-1}= \frac{1}{det(A)}*adj(A)</math> |
| − | *Where <math>adj(A)<math> is the adjoint of <math>A<math>. | + | *Where <math>adj(A)</math> is the adjoint of <math>A</math>. |
*Adjoint is the matrix formed by taking the Transpose of the Co-factor matrix of the original matrix. | *Adjoint is the matrix formed by taking the Transpose of the Co-factor matrix of the original matrix. | ||
| − | *Also <math>A.A^-1=A^-1.A=I<math>, where <math>I<math> is the identity matrix. | + | *Also <math>A.A^-1=A^-1.A=I</math>, where <math>I</math> is the identity matrix. |
*Non-square matrices do not have inverses. | *Non-square matrices do not have inverses. | ||
*Not all square matrices have inverses. | *Not all square matrices have inverses. | ||
Revision as of 05:06, 1 January 2014
MINVERSE(arr)
- is the array of numeric elements
Description
- This function gives the inverse matrix for the given matrix.
- We have to find a inverse of a matrix then it should satisfy the following conditions
- 1.A matrix must be a square matrix.
- 2.It's determinant not equal to 0.
- Let be the 2x2 matrix with the elements
.
- Then the inverse of matrix is denoted by .
- Now let be the matrix is of order .
- Then the inverse of is
- Where is the adjoint of .
- Adjoint is the matrix formed by taking the Transpose of the Co-factor matrix of the original matrix.
- Also , where is the identity matrix.
- Non-square matrices do not have inverses.
- Not all square matrices have inverses.
- A square matrix which has an inverse is called invertible or non-singular, and a square matrix without an inverse is called non-invertible or singular.
- This function will return the result as error when
1. Any one of the cell is non-numeric or any cell is empty or contain text 2. Suppose number of rows not equal to number of columns
Examples
Matrix A A=(4 3
3 2)
MINVERSE(B5:C6)=(-2 3
3 -4)
MATRIX A A=(3 4
6 8)
MINVERSE(C4:D5)=Null, because its det value is 0. MATRIX A A=(2 3
4 7)
MINVERSE(B4:C5)=(3.5 -1.5
-2 1)
 is the array of numeric elements
is the array of numeric elements be the 2x2 matrix with the elements
be the 2x2 matrix with the elements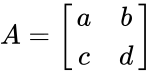 .
.
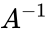 .
.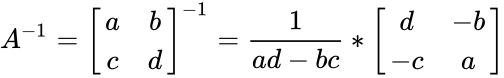
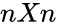 .
.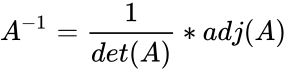
 is the adjoint of
is the adjoint of 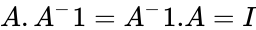 , where
, where 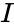 is the identity matrix.
is the identity matrix.