Difference between revisions of "Manuals/calci/EXCHANGE"
Jump to navigation
Jump to search
| Line 6: | Line 6: | ||
*The exchange matrix is the square matrix of a permutation matrix. | *The exchange matrix is the square matrix of a permutation matrix. | ||
*In this matrix the 1 elements reside on the counterdiagonal and all other elements are zero. | *In this matrix the 1 elements reside on the counterdiagonal and all other elements are zero. | ||
| − | *It is a 'row-reversed' or 'column-reversed' version of the identity matrix. | + | *It is a 'row-reversed' or 'column-reversed' version of the identity matrix. |
| + | * Suppose J is an nxn exchange matrix, then the elements of J are defined such that | ||
<math> J_{i,j}=\begin{cases} | <math> J_{i,j}=\begin{cases} | ||
1, j=n-i+1 \\ | 1, j=n-i+1 \\ | ||
| Line 12: | Line 13: | ||
\end{cases}</math>. | \end{cases}</math>. | ||
*It is also called the reversal matrix,backward identity, or standard involutory permutation. | *It is also called the reversal matrix,backward identity, or standard involutory permutation. | ||
| − | *The form of exchange matrices are | + | *The form of exchange matrices are |
| + | <math>J_2=\begin{pmatrix} | ||
| + | 0 & 1 \\ | ||
| + | 1 & 0 | ||
| + | \end{pmatrix}</math> | ||
| + | <math>J_3=\begin{pmatrix} | ||
| + | 0 & 0 & 1 \\ | ||
| + | 0 & 1 & 0 \\ | ||
| + | 0 & 0 & 1 | ||
| + | \end{pmatrix}</math>() | ||
| + | <math> J_n =\begin{pmatrix} | ||
| + | 0 & 0 & \cdots & 0 & 0 & 1 \\ | ||
| + | 0 & 0 & \cdots & 0 & 1 & 0 \\ | ||
| + | 0 & 0 & \cdots & 1 & 0 & 0 \\ | ||
| + | \vdots & \ddots & \vdots \\ | ||
| + | 0 & 1 & \cdots & 0 & 0 & 0 \\ | ||
| + | 1 & 0 & \cdots & 0 & 0 & 0 \\ | ||
| + | \end{pmatrix}</math> | ||
Revision as of 09:51, 27 April 2015
MATRIX("EXCHANGE",order)
- is the order of the Exchange matrix.
Description
- This function gives the exchange matrix of order 3.
- The exchange matrix is the square matrix of a permutation matrix.
- In this matrix the 1 elements reside on the counterdiagonal and all other elements are zero.
- It is a 'row-reversed' or 'column-reversed' version of the identity matrix.
- Suppose J is an nxn exchange matrix, then the elements of J are defined such that
.
- It is also called the reversal matrix,backward identity, or standard involutory permutation.
- The form of exchange matrices are
()
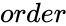 is the order of the Exchange matrix.
is the order of the Exchange matrix.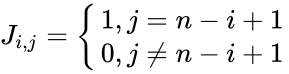 .
.
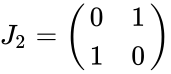
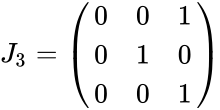 ()
()
