Difference between revisions of "Manuals/calci/BESSELK"
Jump to navigation
Jump to search
| Line 30: | Line 30: | ||
#BESSELK(10,1) = 0.000155369 | #BESSELK(10,1) = 0.000155369 | ||
#BESSELK(2,-1) = NAN | #BESSELK(2,-1) = NAN | ||
| + | |||
| + | ==Related Videos== | ||
| + | |||
| + | {{#ev:youtube|__fdGscBZjI|280|center|BESSEL Equation}} | ||
==See Also== | ==See Also== | ||
Revision as of 13:09, 7 June 2015
BESSELK(x,n)
- Where is the value at which to evaluate the function
- is the integer which is the order of the Bessel Function
Description
- This function gives the value of the modified Bessel function when the arguments are purely imaginary.
- Bessel functions is also called cylinder functions because they appear in the solution to Laplace's equation in cylindrical coordinates.
- Bessel's Differential Equation is defined as:
where is the arbitrary Complex number.
- But in most of the cases α is the non-negative real number.
- The solutions of this equation are called Bessel Functions of order .
- Bessel functions of the first kind, denoted as .
- The Bessel function of the first kind of order can be expressed as:
- The Bessel function of the second kind .
- The Bessel function of the 2nd kind of order can be expressed as:
- So the form of the general solution is .
where: and
are the modified Bessel functions of the first and second kind respectively.
- This function will give the result as error when:
1. or is non numeric 2. , because is the order of the function.
Examples
- BESSELK(5,2) = 0.0040446134
- BESSELK(0.2,4) = 29900.2492
- BESSELK(10,1) = 0.000155369
- BESSELK(2,-1) = NAN
 is the value at which to evaluate the function
is the value at which to evaluate the function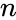 is the integer which is the order of the Bessel Function
is the integer which is the order of the Bessel Function where
where 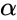 is the arbitrary Complex number.
is the arbitrary Complex number.
 .
.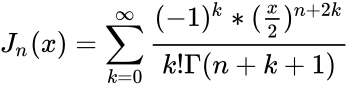
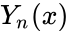 .
.
 .
.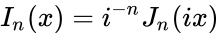 and
and
![{\displaystyle K_{n}(x)=\lim _{p\to n}{\frac {\pi }{2}}\left[{\frac {I_{-p}(x)-I_{p}(x)}{Sin(p\pi )}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/png/15004fa055ca11670b5cc1464033e619ab7f272c)
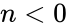 , because
, because