Difference between revisions of "Manuals/calci/NORMSDIST"
Jump to navigation
Jump to search
(Created page with "<div id="6SpaceContent" class="zcontent" align="left"> '''NORMSDIST'''('''x''') '''Where x''' is the value for which the distribution. </div> ---- <div id="1Spa...") |
|||
| (14 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | <div | + | <div style="font-size:30px">'''NORMSDIST (Number,Accuracy)'''</div><br/> |
| + | *<math>Number</math> is the value of the function . | ||
| + | *<math>Accuracy</math> is correct decimal places for the result. | ||
| + | **NORMSDIST(),returns the standard normal cumulative distribution. | ||
| − | + | ==Description== | |
| + | *This function gives the Standard Normal Cumulative Distribution function. | ||
| + | *In Normal Distribution formula, if the Mean is zero and the Standard Deviation is 1, then it is called Standard Normal Distribution. | ||
| + | *The equation for the Standard Normal Density function is:<math> f(x)=\frac{1}{\sqrt 2 \pi}. e^{-{\tfrac{x^2}{2}}}</math> | ||
| + | *This function will return the result as error when the <math>x</math> value is non-numeric. | ||
| − | + | ==Examples== | |
| + | #=NORMSDIST(4.74) = 0.9999975333 | ||
| + | #=NORMSDIST(5.0021) = 0.9999999738 | ||
| + | #=NORMSDIST(1.00006) = 0.8413586589 | ||
| + | #=NORMSDIST(12) = 1.0000002451499 | ||
| − | + | ==Related Videos== | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | {{#ev:youtube|F2rKy2cI8Ss|280|center|NORMSDIST}} | |
| − | + | ==See Also== | |
| + | *[[Manuals/calci/NORMDIST | NORMDIST ]] | ||
| + | *[[Manuals/calci/NORMINV | NORMINV ]] | ||
| + | *[[Manuals/calci/NORMSINV | NORMSINV ]] | ||
| − | + | ==References== | |
| − | + | [http://en.wikipedia.org/wiki/Normal_distribution Normal distribution ] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | *[[Z_API_Functions | List of Main Z Functions]] | |
| − | + | *[[ Z3 | Z3 home ]] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Latest revision as of 17:22, 10 August 2018
NORMSDIST (Number,Accuracy)
- is the value of the function .
- is correct decimal places for the result.
- NORMSDIST(),returns the standard normal cumulative distribution.
Description
- This function gives the Standard Normal Cumulative Distribution function.
- In Normal Distribution formula, if the Mean is zero and the Standard Deviation is 1, then it is called Standard Normal Distribution.
- The equation for the Standard Normal Density function is:
- This function will return the result as error when the value is non-numeric.
Examples
- =NORMSDIST(4.74) = 0.9999975333
- =NORMSDIST(5.0021) = 0.9999999738
- =NORMSDIST(1.00006) = 0.8413586589
- =NORMSDIST(12) = 1.0000002451499
Related Videos
See Also
References
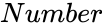 is the value of the function .
is the value of the function .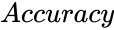 is correct decimal places for the result.
is correct decimal places for the result.
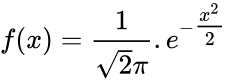
 value is non-numeric.
value is non-numeric.