Difference between revisions of "Manuals/calci/CORRELATIONDATAANALYSIS"
Jump to navigation
Jump to search
| (One intermediate revision by the same user not shown) | |||
| Line 3: | Line 3: | ||
*<math>GroupBy</math> is the group name. | *<math>GroupBy</math> is the group name. | ||
*<math>NewTableFlag</math> is either 0 or 1. | *<math>NewTableFlag</math> is either 0 or 1. | ||
| + | **CORRELATIONDATAANALYSIS(), compares two sets of data. | ||
==Description== | ==Description== | ||
| Line 26: | Line 27: | ||
|ROW2 || 0.9661943464912911 || 1 | |ROW2 || 0.9661943464912911 || 1 | ||
|} | |} | ||
| + | |||
| + | ==Related Videos== | ||
| + | |||
| + | {{#ev:youtube|v=zEXK6M93lb8|280|center|Correlation Analysis}} | ||
==See Also== | ==See Also== | ||
Latest revision as of 17:07, 5 December 2018
CORRELATIONDATAANALYSIS (Array,GroupBy,NewTableFlag)
- is the set of numbers
- is the group name.
- is either 0 or 1.
- CORRELATIONDATAANALYSIS(), compares two sets of data.
Description
- This function shows the correlation data analysis.
- The Correlation coeffiecient shows the relationship between two continuous variables of each other.
- In , is the set of numbers .
- is the group name of correlation and is either "0" or"1".
- We can use the CORREL function to find the correlation coefficient between two variables.
- This is also called Pearson's correlation coefficient.
- It is a measure of the strength of the association between the two variables.
- The correlation coefficient should not be calculated if the relationship is not linear.
- Positive correlation indicates that both variables increase or decrease together, whereas negative correlation indicates that as one variable increases, so the other decreases, and vice versa.
Examples
- CORRELATIONDATAANALYSIS([[56,56,65,65,50,25,87,44,35],[87,91,85,91,75,28,122,66,58]],"rows",1)
| ROW1 | ROW2 | |
|---|---|---|
| ROW1 | 1 | 0.9661943464912911 |
| ROW2 | 0.9661943464912911 | 1 |
Related Videos
See Also
References
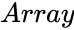 is the set of numbers
is the set of numbers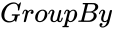 is the group name.
is the group name.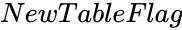 is either 0 or 1.
is either 0 or 1.
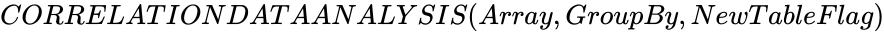 ,
, is the group name of correlation and
is the group name of correlation and 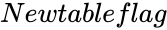 is either "0" or"1".
is either "0" or"1".