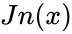Difference between revisions of "Manuals/calci/BESSELJ"
Jump to navigation
Jump to search
| Line 10: | Line 10: | ||
*The solutions of this equation are called Bessel Functions of order n. | *The solutions of this equation are called Bessel Functions of order n. | ||
*Bessel functions of the first kind, denoted as <math>Jn(x)</math> | *Bessel functions of the first kind, denoted as <math>Jn(x)</math> | ||
| − | *The Bessel function of the first kind of order can be expressed as: <math>Jn(x)=\sum_{k=0}^\infity){(-1)^k(x/2)^n+2k}/k!gamma(n+k+1), where gamma(n+k+1)=(n+k)! or *Integral 0 to infinity x^(n+k).e^-x dx. is the gamma function. | + | *The Bessel function of the first kind of order can be expressed as: <math>Jn(x)=\sum_{k=0}^\infity){(-1)^k(x/2)^n+2k}/k!gamma(n+k+1)</math>, where gamma(n+k+1)=(n+k)! or *Integral 0 to infinity x^(n+k).e^-x dx. is the gamma function. |
*This function will give the result as error when 1.x or n is non numeric 2. n<0, because n is the order of the function | *This function will give the result as error when 1.x or n is non numeric 2. n<0, because n is the order of the function | ||
Revision as of 22:20, 1 December 2013
BESSELJ(x,n)
- is the value to evaluate the function
- is the order of the Bessel function and is an integer
Description
- This function gives the value of the modified Bessel function.
- Bessel functions is also called Cylinder Functions because they appear in the solution to Laplace's equation in cylindrical coordinates.
- Bessel's Differential Equation is defined as:
where is the Arbitrary Complex Number.
- But in most of the cases is the non-negative real number.
- The solutions of this equation are called Bessel Functions of order n.
- Bessel functions of the first kind, denoted as
- The Bessel function of the first kind of order can be expressed as: Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Jn(x)=\sum_{k=0}^\infity){(-1)^k(x/2)^n+2k}/k!gamma(n+k+1)} , where gamma(n+k+1)=(n+k)! or *Integral 0 to infinity x^(n+k).e^-x dx. is the gamma function.
- This function will give the result as error when 1.x or n is non numeric 2. n<0, because n is the order of the function
Examples
- BESSELJ(2,3)=0.12894325(EXCEL)Jn(x)=0.10728467204(calci)J1(x)0.5767248079(Actual)J1(x)
- BESSELJ(7,2)=-0.301417224(EXCEL)Jn(x)=NAN(calci)=-0.0046828257(Actual)J1(x)
- BESSELJ(5,1)=-0.327579139(EXCEL)Jn(x)=NAN(calci)
 is the value to evaluate the function
is the value to evaluate the function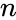 is the order of the Bessel function and is an integer
is the order of the Bessel function and is an integer
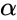 is the Arbitrary Complex Number.
is the Arbitrary Complex Number.