Difference between revisions of "Manuals/calci/XNPV"
Jump to navigation
Jump to search
| Line 14: | Line 14: | ||
*d1 = the 0th payment date | *d1 = the 0th payment date | ||
*Pi = the ith payment. | *Pi = the ith payment. | ||
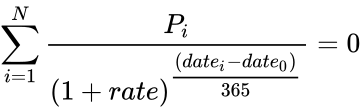
| + | Equation: | ||
| + | <math>\sum_{i=1}^N\dfrac{P_i}{(1+rate)^\tfrac{(date_i-date_0)}{365}}=0</math> | ||
XNPV | XNPV | ||
Revision as of 06:22, 27 February 2014
XNPV(R,V, dates)
- Where 'rate' is the discount rate to apply to the cash flows
- 'V' is a series of cash flows that corresponds to a schedule of payments in dates
- 'dates' is a schedule of payment dates.
Description
- This function computes the net present value for a schedule of cash flows that is not essentially periodic.
- The arguments should be numerics.
- XNPV shows the error value whenever any number in dates precedes the starting date or values and dates contain a different number of values.
- XNPV is calculated as follows:
where:
- di = the ith payment date,
- d1 = the 0th payment date
- Pi = the ith payment.
Equation:
XNPV
Lets see an example,
XNPV(R, V, dates)
B C
-10000 02-01-2009
3000 04-01-2009
4300 11-30-2009
3250 03-15-2010
2200 05-01-2010
?UNIQ4279e6deca12de8c-nowiki-00000002-QINU? is 2069.5268 '
Syntax
Remarks
Examples
Description
| Column1 | Column2 | Column3 | Column4 | |
| Row1 | -10000 | 02-01-2009 | 2069.5268 | |
| Row2 | 3000 | 04-01-2009 | ||
| Row3 | 4300 | 11-30-2009 | ||
| Row4 | 3250 | 03-15-2010 | ||
| Row5 | 2200 | 05-01-2010 | ||
| Row6 | ||||
| Row7 |