Difference between revisions of "Manuals/calci/GFUNCTION"
Jump to navigation
Jump to search
(Created page with "<div style="font-size:30px">'''GFUNCTION (Number)'''</div><br/> *<math>Number</math> is any positive real number. ==Description== *This function shows the value of the Barne...") |
|||
| Line 9: | Line 9: | ||
*According to elementary factors, it is a special case of the double gamma function. | *According to elementary factors, it is a special case of the double gamma function. | ||
*Formally, the Barnes G-function is defined in the following Weierstrass product form: | *Formally, the Barnes G-function is defined in the following Weierstrass product form: | ||
| − | <math>G(1+z)={(2\pi)}^\frac{z}{2}</math> | + | <math>G(1+z)={(2\pi)}^\frac{z}{2}exp(-\frac{z+z^2(1+\gamma)}{2})</math> |
Revision as of 14:54, 8 August 2017
GFUNCTION (Number)
- is any positive real number.
Description
- This function shows the value of the Barnes G-function value.
- In , is any real number.
- is a function that is an extension of super factorials to the complex numbers.
- It is related to the Gamma function, the K-function and the Glaisher–Kinkelin constant, and was named after mathematician Ernest William Barnes.
- According to elementary factors, it is a special case of the double gamma function.
- Formally, the Barnes G-function is defined in the following Weierstrass product form:
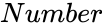 is any positive real number.
is any positive real number.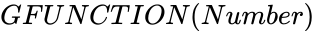 ,
,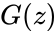 is a function that is an extension of super factorials to the complex numbers.
is a function that is an extension of super factorials to the complex numbers.