Difference between revisions of "Manuals/calci/TTESTEQUALVARIANCES"
Jump to navigation
Jump to search
| Line 9: | Line 9: | ||
*This function calculating the two Sample for equal variances determines whether two sample means are equal. | *This function calculating the two Sample for equal variances determines whether two sample means are equal. | ||
*We can use this test when both: | *We can use this test when both: | ||
| − | *1. | + | *1.The two sample sizes are equal; |
| − | *2. | + | *2.It can be assumed that the two distributions have the same variance. |
| − | *In TTESTTWOSAMPLESEQUALVARIANCES(ar1,ar2,md,alpha,lv), ar1 and ar2 are two arrays of sample values.md is the Hypothesized Mean Difference . | + | *In <math>TTESTTWOSAMPLESEQUALVARIANCES(ar1,ar2,md,alpha,lv), ar1 </math> and <math> ar2 </math> are two arrays of sample values. <math> md </math> is the Hypothesized Mean Difference . |
*Suppose md=0 which indicates that sample means are hypothesized to be equal. | *Suppose md=0 which indicates that sample means are hypothesized to be equal. | ||
| − | *alpha is the significance level which ranges from 0 | + | *<math> alpha </math> is the significance level which ranges from 0 to 1. |
| − | *lv is the logical value like TRUE or FALSE. | + | *<math> lv </math> is the logical value like TRUE or FALSE. |
*TRUE is indicating the result will display in new worksheet.Suppose we are omitted the lv value it will consider the value as FALSE. | *TRUE is indicating the result will display in new worksheet.Suppose we are omitted the lv value it will consider the value as FALSE. | ||
*The t statistic of this function calculated by: | *The t statistic of this function calculated by: | ||
| Line 23: | Line 23: | ||
2.alpha>1 | 2.alpha>1 | ||
3.ar1 and ar2 are having different number of data points. | 3.ar1 and ar2 are having different number of data points. | ||
| − | |||
==Examples== | ==Examples== | ||
Revision as of 03:22, 3 February 2014
TTESTTWOSAMPLESEQUALVARIANCES(ar1,ar2,md,alpha,lv)
- and are set of values.
- is the Hypothesized Mean Difference.
- is the significance level.
- is the logical value.
Description
- This function calculating the two Sample for equal variances determines whether two sample means are equal.
- We can use this test when both:
- 1.The two sample sizes are equal;
- 2.It can be assumed that the two distributions have the same variance.
- In and are two arrays of sample values. is the Hypothesized Mean Difference .
- Suppose md=0 which indicates that sample means are hypothesized to be equal.
- is the significance level which ranges from 0 to 1.
- is the logical value like TRUE or FALSE.
- TRUE is indicating the result will display in new worksheet.Suppose we are omitted the lv value it will consider the value as FALSE.
- The t statistic of this function calculated by:
Failed to parse (Conversion error. Server ("https://wikimedia.org/api/rest_") reported: "Cannot get mml. TeX parse error: Double subscripts: use braces to clarify"): {\displaystyle t={\frac {{\bar {x_{1}}}-{\bar {x_{2}}}}{s_{x}_{1}.s_{x}_{2}.{\sqrt {\frac {2}{n}}}}}}
,where Failed to parse (Conversion error. Server ("https://wikimedia.org/api/rest_") reported: "Cannot get mml. TeX parse error: Double subscripts: use braces to clarify"): {\displaystyle s_{x}_{1}.s_{x}_{2}={\sqrt {\frac {1}{2}}}(s_{x}_{1}^{2}+s_{x}_{2}^{2})}
.
- Here Failed to parse (Conversion error. Server ("https://wikimedia.org/api/rest_") reported: "Cannot get mml. TeX parse error: Double subscripts: use braces to clarify"): {\displaystyle s_{x}_{1}} and Failed to parse (Conversion error. Server ("https://wikimedia.org/api/rest_") reported: "Cannot get mml. TeX parse error: Double subscripts: use braces to clarify"): {\displaystyle s_{x}_{2}} are unbiased estimators of the variances of two samples.Failed to parse (Conversion error. Server ("https://wikimedia.org/api/rest_") reported: "Cannot get mml. TeX parse error: Double subscripts: use braces to clarify"): {\displaystyle s_{x}_{1}.s_{x}_{2}} is the grand standard deviation data 1 and data2 and n is the data points of two data set.
- This function will give the result as error when
1.any one of the argument is nonnumeric. 2.alpha>1 3.ar1 and ar2 are having different number of data points.
Examples
| A | B | C | D | E | F | |
|---|---|---|---|---|---|---|
| 1 | 10 | 15 | 18 | 27 | 12 | 34 |
| 2 | 17 | 20 | 25 | 39 | 9 | 14 |
- =TTESTSAMPLESEQUALVARIANCE(A1:F1,A2:F2,0.5)
See Also
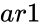 and
and 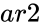 are set of values.
are set of values.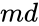 is the Hypothesized Mean Difference.
is the Hypothesized Mean Difference. is the significance level.
is the significance level. is the logical value.
is the logical value.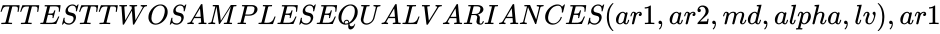 and
and