MATRIX("LEHMER",order)
- is the order of the Lehmer matrix.
Description
- This function gives the lehmer matrix of order 3.
- The the n×n Lehmer matrix, is the constant symmetric matrix defined by Failed to parse (unknown function "\g"): {\displaystyle a_{ij}=\frac{min{i,j}}{max{i,j}} = \begin{cases} \frac{i}{j} & j\ge i \\ \frac{j}{i} & j\g i \end{cases} }
- Also the inverse of a Lehmer matrix is a tridiagonal matrix and is known to be symmetric tridiagonal.
- And the value of this matrix have strictly negative entries (i.e., with positive eigenvalues).
- Example of 2x2 and 3x3 lehmer matrices and its inverses are:
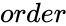 is the order of the Lehmer matrix.
is the order of the Lehmer matrix.