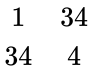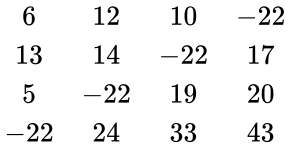Difference between revisions of "Manuals/calci/ANTIDIAGONALWITH"
Jump to navigation
Jump to search
| Line 30: | Line 30: | ||
==Related Videos== | ==Related Videos== | ||
| − | {{#ev:youtube|v=T8ErAYobcbc|280|center| | + | {{#ev:youtube|v=T8ErAYobcbc|280|center|Diagonal Matrix}} |
==See Also== | ==See Also== | ||
Latest revision as of 12:40, 9 April 2019
ANTIDIAGONALWITH()
- Parameter can be any Matrix.
Description
- This function gives the matrix satisfying the anti diagonal properties also it is replacing the constant given number in the anti diagonal entries.
- An anti-diagonal matrix is a matrix where all the entries are zero except those on the diagonal going from the lower left corner to the upper right corner (), known as the anti-diagonal.
- The properties of anti diagonal matrix are:
- 1.The product of two anti-diagonal matrices is a diagonal matrix.
- 2. If A and D are n×n anti-diagonal and diagonal matrices, respectively, then AD,DA are anti-diagonal.
- 3.All anti-diagonal matrices are also persymmetric.
Examples
- ANTIDIAGONALWITH([[1,2],[3,4]],34)
- ANTIDIAGONALWITH([[6,12,10,16],[13,14,15,17],[5,13,19,20],[3,24,33,43]],-22)
Related Videos
See Also
References
 ), known as the anti-diagonal.
), known as the anti-diagonal.